Class 10 Maths Chapter 13 Exercise 13.3

Class 10 Maths Chapter 13 Exercise 13.3 - Surface Areas and Volumes NCERT Solutions

The NCERT Solutions for Class 10 Maths Chapter 13 Exercise 13.3 are designed to help students understand the concepts of “Surface Areas and Volumes.” This chapter, endorsed by CBSE, covers a range of problems related to the surface areas and volumes of various three-dimensional figures, including cubes, cuboids, cylinders, cones, and spheres.

Class 10 Maths Chapter 13 Exercise 13.3 contains questions focused on evaluating volumes, heights, and radii of solids when one solid is melted and recast into another. The solutions are provided by a math expert and are explained in a step-by-step manner, ensuring that students grasp the concepts thoroughly.

In Exercise 13.3, keep these points in mind:

When one metallic object is melted and recast into another, the volume of both objects remains the same.
If earth is removed from a well and used to create a new object, the volume of the well is equivalent to the volume of the new object.
If the speed of water is given (distance per unit time), then the volume of water streamed per unit time can be calculated as: depth × height × speed.

The questions in Exercise 13.3 are crucial for your board examination. We’ve highlighted important and most important questions to help you focus your study efforts.

Get your hands on the Class 10 Maths Chapter 13 Exercise 13.3 – NCERT Solutions PDF for Surface Areas and Volumes. This essential resource includes all the detailed solutions for Chapter 13, making it easier for you to tackle your homework and prepare effectively for exams. The PDF format ensures that you can study offline, giving you the flexibility to access the solutions anytime, anywhere.

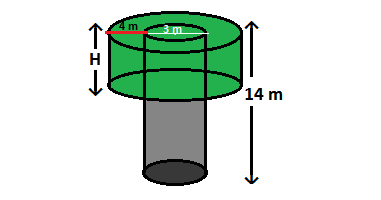
Q1. A metallic sphere of radius 4.2 cm is melted and recast into the shape of a cylinder of radius 6 cm. Find the height of the cylinder. [Important]

Solution. We are given the radius of the sphere = 4.2 cm

Radius of the cylinder = 6 cm

Let the height of cylinder = h

The volume of sphere = Volume of cylinder

Volume of sphere = $\boldsymbol{\frac{4}{3}\pi r^{3}}$ and Volume of cylinder is = $\boldsymbol{\pi r^{2}h}$

$\boldsymbol{\frac{4}{3}\pi \left ( 4.2 \right )^{3}=\pi \left ( 6 \right )^{2}h}$

$\boldsymbol{\frac{4}{3}\times 4.2\times 4.2\times 4.2=36h}$

$\boldsymbol{h=\frac{4}{3}\times 4.2\times 4.2\times 4.2\times \frac{1}{36}}$

h = 2.74

Hence the height of the cylinder = 2.74 cm

Q2. Metallic spheres of radii 6 cm, 8 cm and 10 cm, respectively, are melted to form a single solid sphere. Find the radius of the resulting sphere. [Important]

Solution. We are given the radii of three metalic spheres 6 cm, 8 cm and 10 cm respectively

Sum of the volumes of metalic spheres = Volume of a single solid sphere

The volume of sphere = $\boldsymbol{\frac{4}{3}\pi r^{3}}$

Let the radius of the single solid sphere = r

$\boldsymbol{\frac{4}{3}\pi r^{3}=\frac{4}{3}\pi \left ( 6 \right )^{3}+\frac{4}{3}\pi \left ( 8 \right )^{3}+\frac{4}{3}\pi \left ( 10 \right )^{3}}$

$\boldsymbol{\frac{4}{3}\pi r^{3}=\frac{4}{3}\pi \left ( 6^{3}+8^{3}+10^{3} \right )}$

r ³ = 6³ + 8³ + 10³

r³ = 216 + 512+1000

r³ = 1728

$\boldsymbol{r=\sqrt[3]{1728}=12}$

Hence the radius of single solid sphere is 12 cm

Q3. A 20 m deep well with a diameter of 7 m is dug and the earth from digging is evenly spread out to form a platform 22 m by 14 m. Find the height of the platform. [Very Important]

Solution. We are given the depth of the well, h = 20 m, radius, r of the well = 7/2= 3.5 cm

Let the height of the platform made by the earth taken out from the well =H

The shape of well is the shape of a cylinder

The volume of well = The volume of the earth taken out from the well= Volume of the platform = πr²h =22/7 ×3.5²×20 =22×0.5×3.5×20 = 770 m³

The volume of plateform = Length ×Breadth × Height = 22 × 14 × H

Therefore

22 ×14 H = 770

$\boldsymbol{H=\frac{770}{22\times 14}}$

H = 2.5 m

Hence the height of the plateform = 2.5 m↓

Q4. A well of diameter 3 m is dug 14 m deep. The earth taken out of it has been spread evenly all around it in the shape of a circular ring of width 4 m to form an embankment. Find the height of the embankment. [Most Important]

Solution. Let the height of the embankment= H

Radius of well = 3/2 = 1.5 m, height, h =14 m

The volume of well = The volume of the earth taken out from the well= Volume of the embankment

Volume of well =πr²h =π(1.5)²14

Outer radius of embankment =1.5 +4 = 5.5 m

Inner radius of embankment = 1.5 m

Volume of embankment =π(5.5)²H – π(1.5)²H=πH(5.5²- 1.5²)=πH(30.25 -2.25)=28πH

28πH = π(1.5)²14

28H =2.25×14

H = 2.25/2 =1.125

Hence the height of embankment is 1.125 m

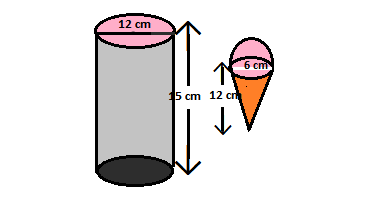
Q5. A container shaped like right circular cylinder having diameter 12 cm and height 15 cm is full of ice-cream .The ice-cream is to be filled into cones of height 12 cm and diameter 6 cm ,having hemisperical shape on the top .Find the number of such cones which can be filled with ice-cream. [Most Important]

Solution.

We are given diameter of right circular cylinder= 12 cm,so radius,r = 12/2 = 6 cm

Height of the cylinder ,h =15 cm

Volume of cylinder = πr²h = π(6)²15 =540π cm³

The volume of ice-cream = Volume of cone + Volume of hemisphere

The height,h of the cone =12 cm and radius of cone and hemisphere,r= 6/2= 3 cm

Volume of cone = $\boldsymbol{\frac{1}{3}\pi r^{2}h}$

$\boldsymbol{\Rightarrow \frac{1}{3}\pi \times 3^{2}\times 12}$

= 3π ×12 = 36π

Volume of hemisphere = $\boldsymbol{\frac{2}{3}\pi r^{3}}$

$\boldsymbol{\Rightarrow \frac{2}{3}\pi \times 3^{3}}$

= 2π× 9 = 18π

∴ The volume of ice-cream = 36π + 18π =54 π cm³

Number of ice-cream cones to be filled

$\boldsymbol{=\frac{Volume \: of \: cylinder}{Volume\: of \: ice-cream \: in\: a\: cone}}$

$\boldsymbol{=\frac{540\pi }{54\pi }=10}$

Hence the number of cones to be filled are 10

Q6.How many silver coins, 1.75 cm in diameter and of thickness 2 mm,must be meted to form a cuboid of dimensions 5.5 cm × 10 cm × 3.5 cm? [Important]

Solution. Let number of coins to be formed = n

Radius of coins,r = 1.75/2 = 0.875 cm and thickness,h=2 mm = 0.2 cm

Volume of coin = πr²h

$\boldsymbol{=\frac{22}{7}\times \left ( 0.875 \right )^{2}\times 0.2}$

=4.4 × 0.125×0.875=0.48125 cm³

Volume of cuboid = Length× Breadth ×Height =5.5 cm × 10 cm × 3.5 cm =192.5 cm³

Number of coins to be melted

$\boldsymbol{=\frac{Volume\: of \: cuboid}{Volume \: of\: a\: coin}}$

$\boldsymbol{=\frac{192.5}{0.48125}}$

= 400

Hence the number of coins to be melted are 400

Q7. A cylindrical bucket ,32 cm high and with radius of base 18 cm ,is filled with sand .This bucket is emptied on the ground and a conical heap of sand is formed .If the height of conical heap is 24 cm,find the radius and slant height of the heap. [Very Important]

Solution. The volume of the bucket = Volume of conical heap

We are given the height of the cylindrical bucket,h = 32 cm and radius of base, = 18 cm

Volume of cylndrical bucket = πr²h = π(18)²32

The height of conical heap =24 cm

Volume of conical heap = $\boldsymbol{\frac{1}{3}\pi r^{2}h}$

The volume of the bucket = Volume of conical heap

$\boldsymbol{\frac{1}{3}\pi \left ( r \right )^{2}24= \pi \left ( 18 \right )^{2}\times 32}$

$\boldsymbol{r^{2}=\frac{18\times 18\times 32\times 3}{24}}$

r² = (18 ×18× 4)

r²=9 ×2×9×2×4

r = 9×2×2= 36 cm

Hence radius of the conical heap = 36 cm

Slant height of the conical heap,l =√(r² +h²) = √(36² + 24²)=√(1296 +576)=√(1872)=12√13 cm

Q8.Water in a canal, 6 m wide and 1.5 m deep, is flowing with a speed of 10 km/h. How much area will it irrigate in 30 minutes, if 8 cm of standing water is needed? [Most Important]

Solution. Let area to be irrigated A in time 30 minutes=30 ×60=1800 seconds

Standing water in the area= 8 cm= o.08 m

We are given width of canal is =6 m and depth=1.5 m

The speed of water =10 km/h = (10×1000)/(60×60) =25/9 m/s

Volume of water flowed in the area in per second =Width ×Depth ×Speed of water=6×1.5×25/9=2×0.5×25=25 m³/s

In 30 minutes (1800 s) the water flowed in the area =25 ×1800=45000 m³

The volume of standing water in 30 minutes = A×h =0.08 A

0.08 A = 45000

A = 45000/0.08= 562500 m²

Q9.A farmer connects a pipe of internal diameter 20 cm from a canal into a cylindrical tank in her field, which is 10 m in diameter and 2 m deep. If water flows through the pipe at the rate of 3 km/h, in how much time will the tank be filled? [Most Important]

Solution. We are given the diameter of the pipe = 20 cm = 0.20 m, so its radius =.20/2=0.10 m

The speed of water in the pipe = 3 km/h = 3000/(60×60) =3000/3600=(5/6) m/s

The volume of water flowed per second through the pipe=πr²h=π(0.10)²×5/6=0.05π/6 m³/s

The diameter of cylindrical tank = 10 m,so its radius =10/2= 5m

Depth(h) of the tank = 2 m

Volume of the tank =πr²h =π(5²)×2= 50π m³

Time taken by the pipe in filling the tank =(Volume of tank)/(Volume of water flowed per second inside the pipe) = 50π /(0.05π/6) =300/0.05= 6000 seconds=100 minutes= 1h 40 minutes

NCERT Solutions of Class 10 Maths

Chapter 1-Real number	Chapter 9-Some Application of Trigonometry
Chapter 2-Polynomial	Chapter 10-Circles
Chapter 3-Linear equations	Chapter 11- Construction
Chapter 4- Quadratic equations	Chapter 12-Area related to circle
Chapter 5-Arithmetic Progression	Chapter 13-Surface areas and Volume
Chapter 6-Triangle	Chapter 14-Statistics
Chapter 7- Co-ordinate geometry	Chapter 15-Probability
Chapter 8-Trigonometry

NCERT Solutions of Class 10 Science

Chapter 1- Chemical reactions and equations	Chapter 9- Heredity and Evolution
Chapter 2- Acid, Base and Salt	Chapter 10- Light reflection and refraction
Chapter 3- Metals and Non-Metals	Chapter 11- Human eye and colorful world
Chapter 4- Carbon and its Compounds	Chapter 12- Electricity
Chapter 5-Periodic classification of elements	Chapter 13-Magnetic effect of electric current
Chapter 6- Life Process	Chapter 14-Sources of Energy
Chapter 7-Control and Coordination	Chapter 15-Environment
Chapter 8- How do organisms reproduce?	Chapter 16-Management of Natural Resources