Skip to content 
Class 9 Maths Chapter 13 Exercise 13.4 - Surface Areas and Volumes NCERT Solutions
NCERT Solutions for Class 9 Maths Exercise 13.4 Chapter 13: Surface Areas and Volumes are designed to enhance the preparation of Class 9 students for the Term 2 CBSE Board exam. These solutions focus on helping students grasp the fundamental concepts required to solve questions in the maths paper. While Chapter 13 covers problems related to the surface areas and volumes of three-dimensional figures, Exercise 13.4 specifically deals with the surface area of spheres.
Practicing these NCERT Solutions for Class 9 Maths Exercise 13.4 will improve students’ math skills, making it one of the most valuable study resources. The solutions are crafted according to CBSE norms, incorporating questions from the NCERT syllabus. The primary goal of solving these questions is to empower students to perform well in both the first and second term CBSE Board exams.
By working through these solutions, students will gain confidence and be better prepared to face their exams. Our detailed collection of questions and answers, created by subject experts and experienced teachers, is designed to help students score high. Each solution includes logical explanations, ensuring that students understand the concepts more effectively, ultimately boosting their performance in the exams.
Q1. Find the surface area of a sphere of radius:
(i) 10.5cm (ii) 5.6cm (iii) 14cm
(Assume π=22/7)
Solution. Surface area of the sphere = 4πr²
(i) Radius (r) of the sphere = 10.5 cm
Surface area of the sphere
= 4 ×(22/7) ×10.5 ×10.5
= 4 ×22 ×1.5 ×10.5
=1386
Hence surface area of the sphere is 1386 cm²
(ii) Radius (r) of the sphere = 5 .6cm
Surface area of the sphere
= 4 ×(22/7) ×5.6 ×5.6
= 4 ×22 ×.8 ×5.6
=394.24
Hence surface area of the sphere is 394.24 cm²
(iii) Radius (r) of the sphere = 14cm
Surface area of the sphere
= 4 ×(22/7) ×14 ×14
= 4 ×22 ×2 ×14
=2464
Hence surface area of the sphere is 2464 cm²
Q2.Find the surface area of a sphere of diameter:
(i) 14cm (ii) 21cm (iii) 3.5cm
(Assume π = 22/7)
Solution: (i) Diameter of the sphere is 14 cm
Therefore radius(r) of the sphere =14/2 = 7 cm
Surface area of the sphere
= 4πr²
Surface area of the sphere with radius 7 cm
= 4 ×(22/7) ×7 ×7
= 4 ×22 ×7
=616
Hence surface area of the sphere is 616 cm²
(ii) Diameter of the sphere is 21 cm
Therefore radius(r) of the sphere =21/2 = 10.5 cm
The surface area of the sphere
= 4πr²
The surface area of the sphere
= 4 ×(22/7) ×10.5 ×10.5
= 4 ×22 ×1.5 ×10.5
=1386
Hence the surface area of the sphere is 1386 cm²
(iii) Diameter of the sphere is 3.5cm
Therefore radius(r) of the sphere =3.5/2 = 1.75 cm
The surface area of the sphere
= 4πr²
The surface area of the sphere
= 4 ×(22/7) ×1.75 ×1.75
= 4 ×22 ×0.25 ×1.75
=38.5
Hence the surface area of the sphere is 38.5 cm²
Q3. Find the total surface area of a hemisphere of radius 10 cm. [Use π=3.14]
Solution: Radius(r) of the hemisphere =10 cm
The total surface area of the hemisphere
= 3πr²
The surface area of the sphere
= 3 ×3.14 ×10 ×10
= 3×314
=942
Hence the surface area of the sphere is 942 cm²
Q4.The radius of a spherical balloon increases from 7cm to 14cm as air is being pumped into it. Find the ratio of surface areas of the balloon in the two cases.
Solution: The surface area of the spherical balloon is =4πr²
When the radius (r) of the balloon was 7 cm then
The surface area of the balloon was = 4π×7×7
When the radius (r) of the balloon was 14 cm then
The surface area of the balloon was = 4π×14×14
The ratio of surface areas of the balloon in the two cases
= (4π×7×7)/(4π×14×14)
= 1/4
Hence the ratio of surface areas of the balloon in the two cases is 1 : 4
Q5. A hemispherical bowl made of brass has inner diameter 10.5cm. Find the cost of tin-plating it on the inside at the rate of Rs 16 per 100 cm2. (Assume π = 22/7)
Solution: The inner diameter of the hemispherical bowl is given 10.5 cm,therefore the radius(r) =10.5/2 =5.25 cm
Inner curved surface area of the hemispherical bowl 44×0.75 ×5.25
=2πr²
=2×(22/7) ×5.25 ×5.25
=44×0.75 ×5.25
= 173.25
Therefore inner curved surface area of the bowl is 173.25 cm²
The rate of tin plating is Rs 16 per 100 cm²
The cost of tinplating inside hemispherical bowl is
=(16/100) × 173.25
= 16×1.7325
=27.72
Hence the cost of tinplating inside hemispherical bowl is Rs. 27.72
Q6. Find the radius of a sphere whose surface area is 154 cm2. (Assume π = 22/7)
Solution: Surface area of the sphere = 4πr²
The given surface area of the sphere is 154 cm²
4πr² = 154
4×(22/7) ×r²= 154
r² = (154 ×7)/(4×22)
r² = (7×7)/4
r = 7/2 =3.5
Hence the radius of the sphere is 3.5 cm
Q7. The diameter of the Moon is approximately one-fourth of the diameter of the Earth. Find the ratio of their surface areas.
Solution: Let the diameter of the earth be x, then the radius of the earth is x/2
The diameter of the moon is
=(1/4) of the earth’s diameter
=(1/4) of x = x/4⇒radius of the moon is x/8
Surface area of the earth is
= 4π(x/2)²=πx²
Surface area of the moon= 4πr² =4π(x/8)² =πx²/16
Ratio of the surface area of the moon and the earth
= πx²/16 ÷ πx²
= 1/16
Hence the ratio of surface areas between moon and the earth is 1 :16
Q8. A hemispherical bowl is made of steel, 0.25 cm thick. The inner radius of the bowl is 5 cm. Find the outer curved surface area of the bowl.
Solution: The inner radius of a hemispherical bowl is 5 cm
The thickness of the hemispherical bowl is 0.25 cm
The radius of the outer curved surface of the hemispherical bowl is = 5 + o.25 =5.25 cm
The outer curved surface area of the bowl
= 2π(Radius of the outer curved surface)²
=2(22/7)×5.25²
=2(22/7)×5.25×5.25
=44×0.75×5.25
=173.25
Hence outer curved surface area of the bowl is 173.25 cm²
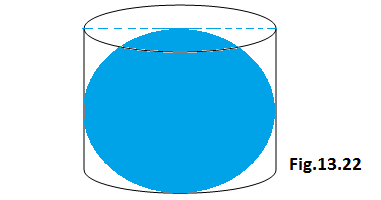
Q9. A right circular cylinder just encloses a sphere of radius r (see fig. 13.22). Find
(i) surface area of the sphere,
(ii) curved surface area of the cylinder,
(iii) ratio of the areas obtained in(i) and (ii).
Solution: It is given that a right circular cylinder just encloses a sphere of radius r
The height(h) of the sphere is = diameter of the sphere =2r
(i) Surface area of the sphere,S =4πr²
(ii) Radius(r) of the sphere = radius of the cylinder(r)
Curved surface area of the cylinder,S’
= 2πrh
=2πr(2r)
S’=4πr²
(iii) The ratio between surface areas of sphere(S) and cylinder(S’)
S/S’ = 4πr²/4πr² =1/1
Hence the ratio between the surface areas of sphere and the curved surface area of the cylinder
S : S’ = 1 : 1
Understanding Class 9 Maths Chapter 13 Exercise 13.4 is important for getting the hang of Surface Areas and Volumes. Our simple, step-by-step solutions for Class 9 Maths Chapter 13 Exercise 13.4 will help you work through each problem easily. With these solutions, Surface Areas and Volumes will feel much easier to learn. Be sure to download the PDF for Class 9 Chapter 13 Maths Exercise 13.4, so you can practice anytime and keep these key concepts handy. Keep practicing, and you’ll do great in your exams!
NCERT Solutions of class 9 maths
| Chapter 1- Number System | Chapter 9-Areas of parallelogram and triangles |
| Chapter 2-Polynomial | Chapter 10-Circles |
| Chapter 3- Coordinate Geometry | Chapter 11-Construction |
| Chapter 4- Linear equations in two variables | Chapter 12-Heron’s Formula |
| Chapter 5- Introduction to Euclid’s Geometry | Chapter 13-Surface Areas and Volumes |
| Chapter 6-Lines and Angles | Chapter 14-Statistics |
| Chapter 7-Triangles | Chapter 15-Probability |
| Chapter 8- Quadrilateral |
NCERT Solutions of class 9 science
NCERT Solutions for Class 9 Maths Exercise 13.4 Chapter 13 Surface Areas and Volumes-Term 2 CBSE Board Exam
NCERT Solutions for Class 9 Maths Exercise 13.4 Chapter 13-Surface Areas and Volumes created here for the purpose of boosting the preparation of class 9 students in maths paper of term 2 CBSE Board exam.NCERT Solutions for Class 9 Maths Exercise 13.4 Chapter 13-Surface Areas and Volumes will help you in clearing the basic concepts of maths that require solving the questions of maths paper in term 2 CBSE Board exam, although chapter 13 is based on problems related to Surface areas and Volumes of three-dimensional figures here this exercise accommodates the surface area of the sphere only. Students can practice and improve their math abilities by tackling the NCERT Solutions.NCERT Solutions is one of the greatest insightful study materials for Class 9 Maths that is created here. These NCERT Solutions incorporate questions from the chapters given in the NCERT Course study according to the CBSE norms. The primary point in solving these questions is to empower the students to score well in Class 9 first and second term CBSE Board exams. NCERT Solutions for Class 9 Maths Chapter13–Surface Areas and Volumes Exercise 13.4 assists students with scoring great and furthermore to confront the exams all the more unhesitatingly, as they gain confidence in tackling these exercises. Click for online shopping Future Study Point.Deal: Cloths, Laptops, Computers, Mobiles, Shoes etc We present to you an itemized assortment of questions and solutions from the exercises with applicable responses, made by our subject specialists and experienced teachers. NCERT solutions plan is to assist students with scoring high in the first and second-term exams. We give appropriate logic and clarifications in the solution of the questions, so students can comprehend the ideas in a superior way.
NCERT Solutions for Class 9 Maths Chapter 13 Surface Areas and Volumes (Term 2 )CBSE Board exam
Class 9 Maths Exercise-13.1 Class 9 Maths Exercise-13.2 Class 9 Maths Exercise-13.3 Class 9 Maths Exercise -13.5 Class 9 Maths Exercise -13.7 Class 9 Maths Exercise -13.8 Class 9 Maths Exercise 13.9 NCERT Solutions Class 9 Maths-All ChaptersNCERT Solutions for Class 9 Maths Exercise 13.4 Chapter 13-Surface Areas and Volumes
Q1. Find the surface area of a sphere of radius:
(i) 10.5cm (ii) 5.6cm (iii) 14cm
(Assume π=22/7)
Ans. Surface area of the sphere = 4πr² (i) Radius (r) of the sphere = 10.5 cm Surface area of the sphere = 4 ×(22/7) ×10.5 ×10.5 = 4 ×22 ×1.5 ×10.5 =1386 Hence surface area of the sphere is 1386 cm² (ii) Radius (r) of the sphere = 5 .6cm Surface area of the sphere = 4 ×(22/7) ×5.6 ×5.6 = 4 ×22 ×.8 ×5.6 =394.24 Hence surface area of the sphere is 394.24 cm² (iii) Radius (r) of the sphere = 14cm Surface area of the sphere = 4 ×(22/7) ×14 ×14 = 4 ×22 ×2 ×14 =2464 Hence surface area of the sphere is 2464 cm²Q2.Find the surface area of a sphere of diameter:
(i) 14cm (ii) 21cm (iii) 3.5cm
(Assume π = 22/7)
Ans.(i) Diameter of the sphere is 14 cm Therefore radius(r) of the sphere =14/2 = 7 cm Surface area of the sphere = 4πr² Surface area of the sphere with radius 7 cm = 4 ×(22/7) ×7 ×7 = 4 ×22 ×7 =616 Hence surface area of the sphere is 616 cm² (ii) Diameter of the sphere is 21 cm Therefore radius(r) of the sphere =21/2 = 10.5 cm The surface area of the sphere = 4πr² The surface area of the sphere = 4 ×(22/7) ×10.5 ×10.5 = 4 ×22 ×1.5 ×10.5 =1386 Hence the surface area of the sphere is 1386 cm² (iii) Diameter of the sphere is 3.5cm Therefore radius(r) of the sphere =3.5/2 = 1.75 cm The surface area of the sphere = 4πr² The surface area of the sphere = 4 ×(22/7) ×1.75 ×1.75 = 4 ×22 ×0.25 ×1.75 =38.5 Hence the surface area of the sphere is 38.5 cm² Q3.Find the total surface area of a hemisphere of radius 10 cm. [Use π=3.14] Radius(r) of the hemisphere =10 cm The total surface area of the hemisphere = 3πr² The surface area of the sphere = 3 ×3.14 ×10 ×10 = 3×314 =942 Hence the surface area of the sphere is 942 cm² Q4.The radius of a spherical balloon increases from 7cm to 14cm as air is being pumped into it. Find the ratio of surface areas of the balloon in the two cases. Ans. The surface area of the spherical balloon is =4πr² When the radius (r) of the balloon was 7 cm then The surface area of the balloon was = 4π×7×7 When the radius (r) of the balloon was 14 cm then The surface area of the balloon was = 4π×14×14 The ratio of surface areas of the balloon in the two cases = (4π×7×7)/(4π×14×14) = 1/4 Hence the ratio of surface areas of the balloon in the two cases is 1 : 4 Q5.A hemispherical bowl made of brass has inner diameter 10.5cm. Find the cost of tin-plating it on the inside at the rate of Rs 16 per 100 cm2. (Assume π = 22/7) Ans.The inner diameter of the hemispherical bowl is given 10.5 cm,therefore the radius(r) =10.5/2 =5.25 cm Inner curved surface area of the hemispherical bowl 44×0.75 ×5.25 =2πr² =2×(22/7) ×5.25 ×5.25 =44×0.75 ×5.25 = 173.25 Therefore inner curved surface area of the bowl is 173.25 cm² The rate of tin plating is Rs 16 per 100 cm² The cost of tinplating inside hemispherical bowl is =(16/100) × 173.25 = 16×1.7325 =27.72 Hence the cost of tinplating inside hemispherical bowl is Rs. 27.72 Q6.Find the radius of a sphere whose surface area is 154 cm2. (Assume π = 22/7) Ans.Surface area of the sphere = 4πr² The given surface area of the sphere is 154 cm² 4πr² = 154 4×(22/7) ×r²= 154 r² = (154 ×7)/(4×22) r² = (7×7)/4 r = 7/2 =3.5 Hence the radius of the sphere is 3.5 cm Q7.The diameter of the Moon is approximately one-fourth of the diameter of the Earth. Find the ratio of their surface areas. Ans. Let the diameter of the earth be x, then the radius of the earth is x/2 The diameter of the moon is =(1/4) of the earth’s diameter =(1/4) of x = x/4⇒radius of the moon is x/8 Surface area of the earth is = 4π(x/2)²=πx² Surface area of the moon= 4πr² =4π(x/8)² =πx²/16 Ratio of the surface area of the moon and the earth = πx²/16 ÷ πx² = 1/16 Hence the ratio of surface areas between moon and the earth is 1 :16 Q8.A hemispherical bowl is made of steel, 0.25 cm thick. The inner radius of the bowl is 5 cm. Find the outer curved surface area of the bowl. Ans. The inner radius of a hemispherical bowl is 5 cm The thickness of the hemispherical bowl is 0.25 cm The radius of the outer curved surface of the hemispherical bowl is = 5 + o.25 =5.25 cm The outer curved surface area of the bowl = 2π(Radius of the outer curved surface)² =2(22/7)×5.25² =2(22/7)×5.25×5.25 =44×0.75×5.25 =173.25 Hence outer curved surface area of the bowl is 173.25 cm²Q9. A right circular cylinder just encloses a sphere of radius r (see fig. 13.22). Find
(i) surface area of the sphere,
(ii) curved surface area of the cylinder,
(iii) ratio of the areas obtained in(i) and (ii).
Ans. It is given that a right circular cylinder just encloses a sphere of radius r
The height(h) of the sphere is = diameter of the sphere =2r
(i) Surface area of the sphere,S =4πr²
(ii) Radius(r) of the sphere = radius of the cylinder(r)
Curved surface area of the cylinder,S’ = 2πrh =2πr(2r) S’=4πr² (iii) The ratio between surface areas of sphere(S) and cylinder(S’)
S/S’ = 4πr²/4πr² =1/1
Hence the ratio between the surface areas of sphere and the curved surface area of the cylinder
S : S’ = 1 : 1
After studying NCERT Solutions for Class 9 Maths Exercise 13.4 Chapter 13 Surface Areas and Volumes-Term 2 CBSE Board Exam, tell us how did you like it. Don’t hesitate to write your views in the comment box that encourages us for improving ourselves.
You can compensate us by donating any amount of money for our survival Our Paytm No 9891436286
NCERT Solutions of Science and Maths for Class 9,10,11 and 12
NCERT Solutions of class 9 maths
| Chapter 1- Number System | Chapter 9-Areas of parallelogram and triangles |
| Chapter 2-Polynomial | Chapter 10-Circles |
| Chapter 3- Coordinate Geometry | Chapter 11-Construction |
| Chapter 4- Linear equations in two variables | Chapter 12-Heron’s Formula |
| Chapter 5- Introduction to Euclid’s Geometry | Chapter 13-Surface Areas and Volumes |
| Chapter 6-Lines and Angles | Chapter 14-Statistics |
| Chapter 7-Triangles | Chapter 15-Probability |
| Chapter 8- Quadrilateral |
NCERT Solutions of class 9 science
CBSE Class 9-Question paper of science 2020 with solutions CBSE Class 9-Sample paper of science CBSE Class 9-Unsolved question paper of science 2019NCERT Solutions of class 10 maths
CBSE Class 10-Question paper of maths 2021 with solutions CBSE Class 10-Half yearly question paper of maths 2020 with solutions CBSE Class 10 -Question paper of maths 2020 with solutions CBSE Class 10-Question paper of maths 2019 with solutionsNCERT solutions of class 10 science
Solutions of class 10 last years Science question papers
CBSE Class 10 – Question paper of science 2020 with solutions CBSE class 10 -Latest sample paper of scienceNCERT solutions of class 11 maths
| Chapter 1-Sets | Chapter 9-Sequences and Series |
| Chapter 2- Relations and functions | Chapter 10- Straight Lines |
| Chapter 3- Trigonometry | Chapter 11-Conic Sections |
| Chapter 4-Principle of mathematical induction | Chapter 12-Introduction to three Dimensional Geometry |
| Chapter 5-Complex numbers | Chapter 13- Limits and Derivatives |
| Chapter 6- Linear Inequalities | Chapter 14-Mathematical Reasoning |
| Chapter 7- Permutations and Combinations | Chapter 15- Statistics |
| Chapter 8- Binomial Theorem | Chapter 16- Probability |
NCERT solutions of class 12 maths
| Chapter 1-Relations and Functions | Chapter 9-Differential Equations |
| Chapter 2-Inverse Trigonometric Functions | Chapter 10-Vector Algebra |
| Chapter 3-Matrices | Chapter 11 – Three Dimensional Geometry |
| Chapter 4-Determinants | Chapter 12-Linear Programming |
| Chapter 5- Continuity and Differentiability | Chapter 13-Probability |
| Chapter 6- Application of Derivation | CBSE Class 12- Question paper of maths 2021 with solutions |
| Chapter 7- Integrals | |
| Chapter 8-Application of Integrals |
