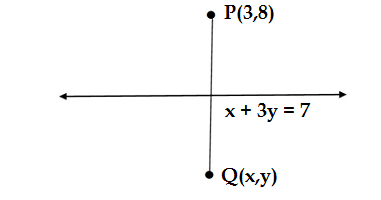Skip to content 
NCERT Solutions for Class 11 Maths Miscellaneous Exercise Straight Lines
NCERT Solutions for Class 11 Maths Miscellaneous Exercise Straight Lines are created by an expert of Future Study Point for helping the online students for doing their homework and preparation of class 11 maths exam. NCERT Solutions for Class 11 Maths Miscellaneous Exercise Straight Lines are the extract of all the exercises from 10.1 to 10.3 of the chapter 10,so it is most important exercise for the preparation of class 11 maths exam of CBSE board.
Q1.Find the values of k for which the line (k -3)x -(4 -k²)y +k²-7k +6 = 0 is
(a) Parallel to the x axis
(b)Parallel to the y axis
(c)Passing through the origin
Click for online shopping
Future Study Point.Deal: Cloths, Laptops, Computers, Mobiles, Shoes etc
Ans. The given line is (k -3)x -(4 -k²)y +k²-7k +6 = 0
-(4 -k²)y =-(k -3)x -k² +7k -6
-(4 -k²)y =-(k -3)x -k² +7k -6
Comparing the equation with y = mx +c
Slope (m) of the given equation is (k-3)/(4 -k²)
The equation of the x axis is y= 0,slope of x axis is 0
We know the slope of two parallel lines are equal to each other
Therefore
(k-3)/(4 -k²) = 0
k -3 = 0
k = 3
Hence if the given line is parallel to x axis then the value of is k =3
Q2. Find the values of θ and p, if the equation x cos θ + y sin θ = p is the normal form of the line √3x + y + 2 = 0
Ans. The given equation is √3x + y + 2 = 0
Comparing it to normal form of the equation x cos θ + y sin θ = p
√3x + y=- 2
-√3 x -y = 2
Dividing both sides by √[(-√3)² +(-1)²]= √(3 +1)=2
Since cos and sin are negative,therefore θ lies in third quadrant
cosθ =cos(π +π/6), sinθ =sin(π +π/6)
cosθ =cos7π/6,sinθ =sin7π/6
θ =7π/6 and p =1
Solutions of Q1 and Q2
Q3.Find the equations of the lines, which cut-off intercepts on the axes whose sum and product are 1 and –6, respectively.
Ans. Let x intercept of the equation is x and y intercept of the equation is b
The sum of both intercept is given 1 and product is -6
a + b = 1….(i)
ab = -6……(ii)
Putting the value of b = (1-a) from equation (i) in equation ( ii)
a(1-a) = -6
a -a² +6 =0
a² -a -6 =0
a² -3a +2a -6=0
a(a -3) +2(a -3) =0
(a -3)(a +2)=0
a=3,-2
For a = 3, b=-2 and for a =-2, b=3
If the x itercept,a and y intercept ,b then equation is written as follows
If a =3 and b= -2 then the equation is as follows
If a =-2 and b= 3 then the equation is as follows
Therefore the required equations are 2x -3y -6=0 and -3x +2y -6 =0
Q4.What are the points on the y-axis whose distance from the line x/3 + y/4 = 1 is 4 units?
Ans. Let the point on y axis is (0,a) whose distance from the line x/3 + y/4 = 1 is 4 units
The given equation is x/3 + y/4 = 1
Writting the given equation into the general form
4x +3y -12 = 0
The distance (d) of a line Ax +By + C = 0 from a point (x1,y1)
The distance of the point (0,a) from the line 4x +3y -12 = 0 ,where A =4, B =3 and C = -12
Since d is given to us = 4 units
20 = ±(3a -12)
In the case 20 = 3a -12⇒ 3a =32 ⇒ a =32/3
In the case 20 =-(3a -12) ⇒20 =-3a +12⇒a =-8/3
Therefore the required points on y axis are (0,32/3) and (0,-8/3)
Q5.Find the perpendicular distance from the origin to the line joining the points
(cos θ,sinθ) and (cos Φ,sinΦ)
Ans. The equation of a straight line passing through a point (x1,y1) is given as follows
(y -y1) = m(x -x1),where m is the slope
The slope of the line(m) joining two points (cos θ,sinθ) and (cos Φ,sinΦ) is as follows
Also the line is passing through (cos θ,sinθ),so x1=cos θ and y1=sin θ
(y -sinθ)(cosΦ -cosθ) = (sinΦ -sinθ)(x -cosθ)
y(cosΦ -cosθ) -sinθ(cosΦ -cosθ) =sinΦ(x -cosθ)-sinθ(x -cosθ)
y(cosΦ -cosθ) -sinθcosΦ +sinθcosθ = xsinΦ -sinΦ cosθ -xsinθ +sinθcosθ
y(cosΦ -cosθ) +x(sinθ -sinΦ) +(sinΦ cosθ-sinθcosΦ) =0
y(cosΦ -cosθ) +x(sinθ -sinΦ) +sin(Φ-θ) = 0
Rearranging into the general form
x(sinθ -sinΦ) +y(cosΦ -cosθ) + sin(Φ-θ) =0
The distance(d) of the point (x1,y1) from the line Ax +By +C =0
Therefore the distance (d) from the origin(0,0) to the line x(sinθ -sinΦ) +y(cosΦ -cosθ) -sin(θ -Φ) =0
Q6.Find the equation of the line parallel to y-axis and drawn through the point of intersection of the lines x – 7y + 5 = 0 and 3x + y = 0.
Ans. A line which is parallel to Y axis creats 90° angle with positive side of X axis
Therefore the slope ,m of the line is = tan 90° = sin 90°/cos 90° =1/0
Also the line is passing through the point of intersection of both lines x – 7y + 5 = 0…(i) and 3x + y = 0…..(ii)
For solving both of the lines ,multiplying equation (i) by 3,we get equation (iii)
3x – 21y + 15 =0…..(iii)
Subtracting equation (iii) from equation (ii)
22y -15 =0
22y = 15
y = 15/22
Putting the value of y in equation (i)
3x + 15/22 = 0
3x = -15/22
x = -15/66 = -5/22
Therefore both of the given lines intersects each other at (15/22,-5/22)
The equation of the line which is passing through a point (x1,y1) with slope m is given by
y – y1=m (x -x1)
The equation of the line which is passing through (15/22,-5/22) with slope 1/0
y – 15/22 = (1/0)(x +5/22)
x + 5/22 =0
x = -5/22
Hence the required equation of the line is -5/22
Q7.Find the equation of a line drawn perpendicular to the line x/4 + y/6 = 1 through the point, where it meets the y-axis.
Ans. Let the given line meets the Y axis at (0,y),wherefrom we have to find out the equation of perpendicular line
Writting the given line x/4 + y/6 = 1 into the x intercept form y = mx +c
3x + 2y = 12
2y = -3x +12
y = (-3/2)x + 6
On comparing the standard form
m = -3/2
We know
The product of slopes of two perpendicular line =-1
Slope of perpendicular line to given line = -1/Slope of the given line= -1/(-3/2) = 2/3
Since the given line is passing through (0,y) ,so putting x =0 in the given equation
y/6 =1
y = 6
The equation of perpendicular line drawn from (0,6) is given as
y -6 = (2/3)( x -0)
3y -18 =2x
2x -3y +18 =0
Hence required equation of the line is 2x -3y +18 =0
Q8. Find the area of the triangle formed by the lines y – x = 0, x + y = 0 and x – k = 0.
Ans. Let’s find the verices of the triangle by solving these equations
The given equations are y – x = 0….(i) x + y = 0….(ii) and x – k = 0…..(iii)
Solving equations (i) and (ii),we get y = 0 and x =0,so one of the vertex is (0,0)
Solving equations (ii) and (iii),we get y = -k and x =k,so one of the vertex is (k,-k)
Solving equations (i) and (iii),we get y = k and x =k,so one of the vertex is (k,k)
The area of the triangle having the vertices (x1,y1)(x2,y2) and (x3,y3) is given as
Area of Δ = (1/2)[ (x1(y2– y3) + x2(y3– y1) +x3(y1– y2) ]
Area of the triangle whose vertices are (0,0),(k,-k) and (k,k)
Area of Δ = (1/2) [0(-k-k) +k(k-0) + k(0 +k)]
=(1/2) [0 + k² + k²]
(1/2) .2k² = k²
Hence area of the required triangle is k² units.
Q9.Find the value of p so that the three lines 3x + y – 2 = 0, px + 2y -3 = 0 and 2x – y -3 = 0 may intersect at one point.
Ans.The given lines are 3x + y – 2 = 0…(i) px + 2y -3 = 0 …..(ii) and 2x – y -3 = 0 ….(iii)
Solving the equations (i) and (iii),we get the point of intersection of (i) and (iii) equations
Adding equation (iii) to equation (i)
5x -5 =0
5x =5
x =1
Putting the value of x in equation (i)
3×1 + y -2 =0
3 + y -2 =0
y +1 =0
y =-1
The point of intersection of (i) and (iii) line is (1,-1),since it is given that three lines intersect on the same point,therefore the point (1,-1) should satisfy the (ii) equation
Putting x =1 and y =-1
p×1+2×-1-3=0
p -2 -3 =0
p-5 =0
p =5
Hence the required value of p is 5
Q10. If three lines whose equations are y = m1x + c1, y = m2x + c2 and y = m3x + c3 are concurrent, then show that m1 (c2 – c3) + m2 (c3 – c1) + m3 (c1 – c2) = 0.
Ans. The given equations are y = m1x + c1…..(i) y = m2x + c2 ……(ii) and y = m3x + c3 …….(iii)
Solving equations (i) and equation (ii),for this,substracting equation (ii) from equation (i)
0 = x(m1– m2) +(c1 -c2)
x = (c2 -c1)/(m1– m2)
Putting the value of x in equation (i)
y = m1x + c1
y = m1 (c2 -c1)/(m1– m2)+ c1
Since lines (i),(ii) and (iii) are concurrent therefore the line (i) and (ii) intersect each other at the points [(c2 -c1)/(m1– m2), m1 (c2 -c1)/(m1– m2)+ c1] should satisfy the equation (iii)
m1 (c2 -c1)/(m1– m2)+ c1=m3 (c2 -c1)/(m1– m2)+c3
m1 (c2 -c1)/(m1– m2)=m3 (c2 -c1)/(m1– m2)+c3-c1
m1 (c2 -c1)=m3 (c2 -c1)+(c3-c1)(m1-m2) =0
m1c2– m1c1 = m3c2– m3c1 + m1c3– m2c3– m1c1 + m2c1
m1c2 = m3c2– m3c1 + m1c3– m2c3+ m2c1
m1c2 -m3c2+m3c1 – m1c3+m2c3 – m2c1=0
m1 (c2 – c3) + m2 (c3 – c1) + m3 (c1 – c2) = 0,Hence proved
Q11.Find the equation of the lines through the point (3, 2) which make an angle of 45° with the line x – 2y = 3.
Ans.Let the slope of the line which is passing through the given point (3,2) =m1
Rewritting the given equation of the line into the slope intercept form y =mx +c
-2y = -x + 3
y = -x/-2 + 3/-2
y =x/2 -3/2
On comparing with y = mx +c
Slope of the line is = 1/2
Let it is m2=1/2
The angle between both lines given to us = 45°
The relationship between slopes and angle between both lines is given as
Putting the values of m1 ,m2 and tan θ
It is written as
tan 45° = ±(1- 2m1)/(2 +m1)
Taking positive root
1 = (1- 2m1)/(2 +m1)
2 +m1= 1- 2m1
3m1 = 1-2 =-1
m1= -1/3
Taking negative root
1=-(1- 2m1)/(2 +m1)
2 +m1 = -1+2m1
m1= 3
Therefore equations of the line passing through the point (3,2) are
y -2 = (-1/3)(x -3) and y -2 = 3(x -3)
3y -6 = -x +3 and y-2 = 3x -9
x +3y -9 =0 and 3x -y -7 =0
Hence required equations of the line are x +3y -9 =0 and 3x -y -7 =0
Q12. Find the equation of the line passing through the point of intersection of the lines 4x + 7y – 3 = 0 and 2x – 3y + 1 = 0 that has equal intercepts on the axes.
Ans. The given equations given to us are 4x + 7y – 3 = 0…(i) and 2x – 3y + 1 = 0….(ii)
Multiplying the equation (ii) by 2,we get equation (iii)
4x -6y +2 =0…(iii)
Substracting equation (iii) from equation (i)
13y -5 = 0
y = 5/13
Putting this value of y in equation (i)
4x + 7 ×5/13 -3 =0
4x = 3 -35/13 = (39 -35)/13 =4/13
x = 1/13
So, point of intersection of both given line is (1/13,5/13)
The required line is passing through (1/13,5/13) with equal interceps of both axis.
Let the both intercepts of the axis are a
Therefore
x/a + y/a = 1
x +y = a
It should satisfy (1/13, 5/13)
1/13 +5/13 =a
a = 6/13
Hence the required equation is
x + y = 6/13
13x + 13y = 6
Q13.Show that the equation of the line passing through the origin and making an angle θ with the line y = mx + c is
Ans. We have to find out equation of the line which is passing through the origin,so supossing its slope m1 the equation is given by
y -0 = m1(x -0)
m1 = y/x
The given equation is y = mx + c,therefore its slope,=m2= m
The relationship between slopes and angle between both lines is given as
Putting the values of m1 ,m2 and tan θ
tan θ = ±(m- y/x)/( 1 + ym/x)
Taking the positive roots
tan θ =(m- y/x)/( 1 + ym/x)
tan θ + (y/x). m tan θ = m – y/x
y/x + (y/x). m tanθ = m – tan θ
(y/x) (1 + m tanθ) = m – tan θ
y/x = (m – tan θ)/( 1 + m tan θ)….(i)
Similarly we can get by taking tan θ = -(m- y/x)/( 1 + ym/x)
y/x = (m + tan θ)/( 1 – m tan θ)….(ii)
From equation (i) and (ii) we get
Q14.In what ratio ,the line joining ,(-1,1) and (5,7) is divided by the line x + y =4.
Ans. Let, the line joining,(-1,1) and (5,7) is divided by the line x +y =4 in the ratio of m : n
So, let’s get point of intersection of the line joining (-1,1) and (5,7) and the line (x +y =4)
The slope of the line joining the given point is ,m =(7-1)/(5+1) =6/6 =1
The equation of the line passing through (-1,1) is given as
(y-1) = 1×(x +1)
y-1 = x+1
y -x =2……(i)
The given equation is x +y =4….(ii)
Solving both equation (i) and (ii),we get y=3 and x=1
Therefore point of intersection of both lines (x +y=4) and (y-x =2) is (1,3)
The point (1,3) divides the line segment joining two points (-1,1) and (5,7) in the ratio of m:n
Applying the section formula
1 = (5m -n)/(m+n)
m +n = 5m -n
4m =2n
m : n = 2 ;4
m : n =1 : 2
Or
3 =(7m +n)/(m+n)
3m +3n = 7m +n
-4m = -2n
m : n = 2 : 4 = 1 : 2
Therefore the given line divides the line segment joining the given points in the ratio of 1 :2
Q15.Find the distance of the line 4x +7y +5=0 from the point (1,2) along the line 2x -y =0.
Ans. The given lines are 4x +7y +5=0 ….(i) and 2x -y =0…..(ii)
Let’s find the distance of equation (i) from the point (1,2) which is on the line 2x -y =0.
The distance of the line (i) from (1,2) =Distance of the point (1,2) and the point of intersection line (i) and (ii)
Therefore solving both equation (i) and (ii)
Multiplying equation (ii) by 2 ,we get equation (iii)
4x -2y =0….(iii)
Substracting equation (ii) from equation (i)
9y +5 =0
y =-5/9
Putting the value of y in equation (ii)
2x -(5/9) =0
2x +5/9 =0
x =-5/18
Therefore point of intersection of the line (i) and (ii) is (-5/18,-5/9)
So, the distance(d) between the point (1,2) and (-5/18,-5/9)
d = √[(1+5/18)²+ (2+5/9)²]
= √[(23/18)²+ (23/9)²]
=√[529/324+ 529/81]
=√(529+ 2116)/324]=√(2645/324) =23√5/18
Hence the required distance of the line 4x +7y +5=0 from the point (1,2) is 23√5/18
Q16.Find the direction in which a straight line must be drawn through the point (-1, 2) so that its point of intersection with the line x + y = 4 may be at a distance of 3 units from this point.
Ans.Let the slope of the straight line m,and its equation is y = mx +c
The line is passing through the point (-1,2)
Since c is a constant so,putting the values x=-1 and y =2
2 = m(-1) +c
c =2 +m
Putting the value of c =2 +m in the equation
y = mx + 2 +m
y = m(x +1) +2…..(i)
The given equation is
x +y =4 …….(ii)
Putting the value x = 4 -y from (ii) in equation (i)
y = m(4-y +1) +2
y = 5m -my +2
y+ my = 5m +2
y(1 +m) = 5m +2
y = (5m +2)/(m+1)
Putting the value of y in equation (ii)
x + (5m +2)/(m+1) =4
x = 4-(5m +2)/(m+1) = (4m+4-5m-2)/(m+1) = (2-m)/(m+1)
So, the point of intersection of both lines is [(2-m)/(m+1),(5m +2)/(m+1)]
The distance between point of intersecton and the given point (-1,2) is given to us 3 units
3 =√[ {(5m +2)/(m+1 ) -2}² +{(2-m)/(m+1) +1}²]
Squaring both sides
{(5m +2)/(m+1 ) -2}² +{(2-m)/(m+1) +1}² =9
(5m+2-2m-2)²/(m+1)² + (2-m+m+1)²/(m+1)² =9
(3m )² + 3² =9
9m² +9 =9
9m² =0
m =0
Hence slope of the line is 0 i.e the line is parallel to x axis
Q17.The hypotenuse of a right-angled triangle has its ends at the points (1, 3) and (-4, 1). Find the equation of the legs (perpendicular sides) of the triangle.
Ans. The given ends of the hypotenuse are (1,3) and (-4,1)
One of side passes through (1,3) and the other passes through (-4,1)
The equation of the line which passes through (x1,y1) is given by
(y – y1) = m(x –x1)
Therefore the line that passes through (1,3) is
y -3 = m(x -1)……(i)
The line that passes through (-4,1) is
y -1 = -1/m(x +4)……(ii)(i.e the product of the slopes of two perpendicular line is -1)
The sides of the tringle can have any slope,so placing the specific value of m i.e m=0
Putting m =0 in equation (i) and in equation (ii)
y -3 = 0 ⇒y= 3
y – 1= -1/0(x +4)
-x -4=0
x =-4
If equation of one side is y=3 then equation of other side is x =-4
Or if the slope of the line (i) is 1/m
y-3 = 1/m(x-1)
y-3 = 1/0(x-1)
x-1=0⇒ x= 1
Then equation of second line is
y -1 =-m(x +4)
y-1 =0
y =1
If equation of one side is x =1 then equation of other side is y =1
Q18.Find the image of the point (3, 8) with respect to the line x + 3y = 7 assuming the line to be a plane mirror.
Ans. Let the image of the point (3,8) is (x,y) with respect to the line x +3y =7 assuming the line to be a plane mirror.
The line x + 3y is the perpendicular bisector of the line segment PQ
The mid point of the line PQ = [(x+3)/2,(8+y)/2]
The mid point of PQ passes through the line x +3y =7,so it will satisfy its coordinates
(x+3)/2 + 3(8+y)/2 =7
x +3 +24 +3y =14
x + 3y + 13=0…….(i)
Since the line PQ ⊥ (x +3y =7)
Therefore product of their slopes = -1
The slope of the given line (y =-x/3+7/3) is -1/3
The slope of PQ = (y-8)/(x -3)
∴ (-1/3)(y-8)/(x-3) =-1
y -8 = 3x -9
3x -y -1 =0…..(ii)
Multiplying equation (i) by 3,we get equation (iii)
3x +9y +39 =0….(iii)
Substracting equation (iii) from equation (ii)
-10y -40 =0
y = -40/10 =-4
Putting the value y =-4 in equation (ii)
3x +4 -1 =0
3x = -3
x = -1
Hence the image of the given point (3,8) with respect to the given line is (-1,4)
Q19. If the lines y = 3x+ 1 and 2y= x + 3 are equally inclined to the line y = mx + 4, find the value of m.
Ans. The given equations are y = 3x+ 1….(i) 2y= x + 3 …..(ii) and y = mx + 4…..(iii)
Rearranging the equations into their slope-intercept form i.e y = mx +c
Slope of equation (i) = 3,Slope of equation (ii) =1/2 and Slope of equation (iii) =m
We are given that
The angle between y=3x +1 and the line y =mx +4 =The angle between 2y = x+3 and the line y = mx +4
(m-3)/(1 +3m) =±(2m-1)/(2 +m)
Taking the positive root
(m-3)/(1 +3m) =(2m-1)/(2 +m)
Cross multiplying it
(m-3)(2 +m) = (1 +3m)(2m -1)
2m +m²-6 -3m = 2m -1 +6m²-3m
5m² +5 = 0
m² +1 = 0
m= √-1
m is not real ,so neglecting this value of m
(m-3)/(1 +3m) =-(2m-1)/(2 +m)
(m-3)(2 +m) = -(1 +3m)(2m -1)
2m +m²-6 -3m = -2m +1 -6m²+3m
7m²-2m -7 =0
Hence the required value of m is (1±5√2)/7
Q20.If sum of the perpendicular distances of a variable point P (x, y) from the lines x + y-5 = 0 and 3x – 2y + 7 = 0 is always 10. Show that P must move on a line.
Let the perpendicular distances of the given lines from the point P(x,y) are d1 and
d1
The perpendicular distance(d) from a point P(x,y) to the given line Ax + By +C is given as
It is given to us that
d1+d1=10
√13x +√13 y -5√13 + 3√2 x -2√2 y +7√2 = 10√26
x(√13 +3√2) + y(√13 -2√2) +7√2-5√13 – 10√26 =0
The equation we have got is the equation of a line,so P(x,y) must move on it
Similarly we get the equation of another line by taking another sign
Q21.Find equation of the line which is equidistant from parallel lines 9x + 6y – 7 = 0 and 3x + 2y + 6 = 0.
Ans. Let the point (x,y) is an arbitrary point which is equidistant from parallel lines 9x +6y -7 =0 and 3x +2y +6 =0
The perpendicular distance(d) from a point (x,y) to the given line Ax + By +C is given as
According to the question
9x +6y -7 = ±3(3x +2y +6)
Taking positive sign
9x +6y -7 = 9x +6y +18
-7 = 18,it is impossible
Taking negative sign
9x +6y -7 = -3(3x +2y +6)
9x +6y -7 = -9x -6y -18
18x +12y +11 =0
Hence the required equation of the line is 18x +12y + 11 =0
Q22. A ray of light passing through the point (1, 2) reflects on the x-axis at point B and the reflected ray passes through the point (5, 3). Find the coordinates of B.
Ans.
Let a ray AB incident on the x axis at the point (a,0) and reflected ray is BC
Drawing a normal BM⊥XX’
∠ABM =Incident angle=Φ, ∠CBM = Reflected angle=Φ(i.e reflected angle =incident angle)
For finding the slope of the incident ray AB,let’s find the angle ∠OBA
∠OBA = 180°-(Φ+Φ+θ)= 180°-(2Φ+θ) = 180°-[2(90-θ)+θ)]= 180°-180 +2θ-θ =θ
∠ABX’ = 180°-θ
Therefore the slope of ray AB is = tan( 180°-θ)=-tanθ
The slope of BC is = tanθ
Since AB is passes through (1,2) and (a,0) and BC passes through (a,0) and (5,3)
-tan θ =(0-2)/(a-1)
tan θ = 2/(a -1)……(i)
tan θ =(3-0)/(5-a)= 3/(5-a)…..(ii)
2/(a-1) = 3/(5-a)
10 -2a =3a -3
5a =13
a = 13/5
Hence the required point on the X-axis is (13/5,0)
Q23. Prove that the product of the lengths of the perpendiculars drawn from the points [√(a²-b²),0] and [-√(a²-b²),0] to the line (x/a)cosθ +(y/b) sinθ =1 is b²
Ans. We know the length of perpendicular (d) drawn from a point (x,y) to the line Ax +By +C =0 is given by
The given line is
(x/a)cosθ +(y/b) sinθ =1
Wriiting it in the standard form
(bcosθ)x + (asinθ)y -ab =0
Let the length of perpendiculars drawn from the given points to the given lines are
d1 and d2
Similarly
= b²
Hence the required product of the perpendiculars is b²
Q24. A person standing the junction (crossing) of two straight paths represented by the equations 2x – 3y + 4 = 0 and 3x + 4y – 5 = 0 wants to reach the path whose equations is 6x – 7y + 8 = 0 in the lease time. Find the equation of the path that he should follow.
Ans. It is given that
2x – 3y + 4 = 0…. (i)
3x + 4y – 5 = 0…. (ii)
6x – 7y + 8 = 0…. (iii)
Here the person is standing at the junction of the paths represented by (i) and (ii)
By solving equations (i) and (ii) we get
x = – 1/17 and y = 22/17
Hence, the person is standing at point (- 1/17, 22/17)
We know that the person can reach path (iii) in the least time if he walks along the perpendicular line to (iii) from point (- 1/17, 22/17)
Here the slope of the line (iii) = 6/7
We get the slope of the line perpendicular to line (iii) = – 1/(6/7) = – 7/6
So the equation of line passing through (- 1/17, 22/17) and having a slope of – 7/6 is written as
By further calculation
6(17y – 22) = – 7(17x + 1)
By multiplication
102y – 132 = – 119x – 7
We get, 1119x + 102y = 125
Therefore, the path that the person should follow is 119x + 102y = 125
You can compensate us by donating any amount of money for our survival
Our Paytm NO 9891436286
NCERT Solutions of Science and Maths for Class 9,10,11 and 12
NCERT Solutions of class 9 maths
| Chapter 1- Number System | Chapter 9-Areas of parallelogram and triangles |
| Chapter 2-Polynomial | Chapter 10-Circles |
| Chapter 3- Coordinate Geometry | Chapter 11-Construction |
| Chapter 4- Linear equations in two variables | Chapter 12-Heron’s Formula |
| Chapter 5- Introduction to Euclid’s Geometry | Chapter 13-Surface Areas and Volumes |
| Chapter 6-Lines and Angles | Chapter 14-Statistics |
| Chapter 7-Triangles | Chapter 15-Probability |
| Chapter 8- Quadrilateral |
NCERT Solutions of class 9 science
CBSE Class 9-Question paper of science 2020 with solutions
CBSE Class 9-Sample paper of science
CBSE Class 9-Unsolved question paper of science 2019
NCERT Solutions of class 10 maths
CBSE Class 10-Question paper of maths 2021 with solutions
CBSE Class 10-Half yearly question paper of maths 2020 with solutions
CBSE Class 10 -Question paper of maths 2020 with solutions
CBSE Class 10-Question paper of maths 2019 with solutions
NCERT solutions of class 10 science
Solutions of class 10 last years Science question papers
CBSE Class 10 – Question paper of science 2020 with solutions
CBSE class 10 -Latest sample paper of science
NCERT solutions of class 11 maths
| Chapter 1-Sets | Chapter 9-Sequences and Series |
| Chapter 2- Relations and functions | Chapter 10- Straight Lines |
| Chapter 3- Trigonometry | Chapter 11-Conic Sections |
| Chapter 4-Principle of mathematical induction | Chapter 12-Introduction to three Dimensional Geometry |
| Chapter 5-Complex numbers | Chapter 13- Limits and Derivatives |
| Chapter 6- Linear Inequalities | Chapter 14-Mathematical Reasoning |
| Chapter 7- Permutations and Combinations | Chapter 15- Statistics |
| Chapter 8- Binomial Theorem | Chapter 16- Probability |
CBSE Class 11-Question paper of maths 2015
CBSE Class 11 – Second unit test of maths 2021 with solutions
NCERT solutions of class 12 maths
| Chapter 1-Relations and Functions | Chapter 9-Differential Equations |
| Chapter 2-Inverse Trigonometric Functions | Chapter 10-Vector Algebra |
| Chapter 3-Matrices | Chapter 11 – Three Dimensional Geometry |
| Chapter 4-Determinants | Chapter 12-Linear Programming |
| Chapter 5- Continuity and Differentiability | Chapter 13-Probability |
| Chapter 6- Application of Derivation | CBSE Class 12- Question paper of maths 2021 with solutions |
| Chapter 7- Integrals | |
| Chapter 8-Application of Integrals |
