NCERT Solutions for Class 9 exercise 15.1 of Chapter 15 Probability

NCERT Solutions for Class 9 exercise 15.1 of Chapter 15 Probability are presented here by an expert of maths, these NCERT Solutions for Class 9 exercise 15.1 of Chapter 15 Probability is an important input for your forthcoming exams. You can clear all your doubts in studying these NCERT solutions of the chapter 15 probability. All questions of exercise 15.1 are created by a step by step method therefore we hope every student will understand each solution. Chapter 15 probability is very easy so it is a good opportunity for the students to score well in the exam by solving all the questions of the probability chapter.

Click for online shopping

Future Study Point.Deal: Cloths, Laptops, Computers, Mobiles, Shoes etc

NCERT Solutions of class 9 maths

Chapter 1- Number System	Chapter 9-Areas of parallelogram and triangles
Chapter 2-Polynomial	Chapter 10-Circles
Chapter 3- Coordinate Geometry	Chapter 11-Construction
Chapter 4- Linear equations in two variables	Chapter 12-Heron’s Formula
Chapter 5- Introduction to Euclid’s Geometry	Chapter 13-Surface Areas and Volumes
Chapter 6-Lines and Angles	Chapter 14-Statistics
Chapter 7-Triangles	Chapter 15-Probability
Chapter 8- Quadrilateral

Q1.In a cricket match, a batswoman hits a boundary 6 times out of 30 balls she plays. Find the probability that she did not hit a boundary.

Ans. The number of balls played by batswoman = 30,so total possible outcomes = 30

She hits number of boundary 6 times,so the number of balls in which she didn’t hit the boundary = 30 – 6 = 24

Therefore the favourabe outcomes = 24

∴P( she didn’t hit boundary) = Favourable outcomes /Total possible outcomes =24/30 =4/5

Q2.1500 families with 2 children were selected randomly, and the following data were recorded

Compute the probability of a family, chosen at random, having
(i) 2 girls
(ii) 1 girl
(iii) no girl
Also, check whether the sum of these probabilities is 1

Ans. Total number of families surveyed =1500, therefore total possible outcomes are 1500

(i) The number of families who have 2 girls= 475,therefore favourable outcomes are 475

∴ P( families who have 2 girls) = 475/1500 =19/60

(ii) The number of families who have 1 girls= 814,therefore favourable outcomes are 814

P( families who have 1 girls)=814/1500 =407/750

(iii)The number of families who have no girls=211,therefore favourable outcomes are 211

P( families who have no girls)=211/1500

The sum of all the probabilty = 19/60 +407/750 +211/1500 =(475 +814 +211)/1500 =1500/1500 = 1

Therefore sum of all the mentioned probabilities is 1

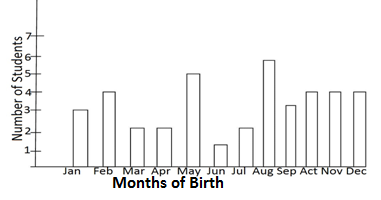
Q3.In a particular section of class IX, 40 students were asked about the month of their birth and the following graph was prepared for the data so obtained.

Find the probability that a student of the class was born in August.

Ans. Total number of students of IX class who were asked their birthday = 40, therefore total possible outcomes are 40

The number of students whoes birthdays lies on august are=6, therefore favourable outcomes are 6.

P(students whose birthday lies on august) = 6/40 = 3/20

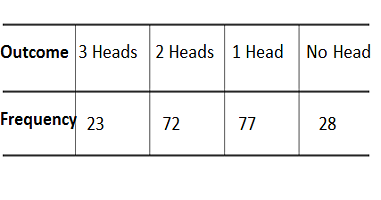
Q4.Three coins are tossed simultaneously 200 times with the following frequencies of different outcomes.

If the three coins are simultaneously tossed again, compute the probability of 2 heads coming up.

Ans. Three coins are tossed simultaneously 200 times,therefore total possible outcomes are 200

Frequencey of the outcomes of 2 head is =72,therefore favourable outcomes are 72

∴P( of 2 heads) = 72/200 = 9/25

Hence required probability is 9/25

Q5.An organisation selected 2400 families at random and surveyed them to determine a relationship between income level and the number of vehicles in a family. The information gathered is listed in the table below.

Suppose a family is chosen. Find the probability that the family chosen is
(i) earning ₹ 10000-13000 per month and owning exactly 2 vehicles.
(ii) earning ₹16000 or more per month and owning exactly 1 vehicle.
(iii) earning less than ₹ 7000 per month and does not own any vehicle.
(iv) earning ₹13000-16000 per month and owning more than 2 vehicles.
(v) owning not more than 1 vehicle.

Ans. Total families surveyed = 2400,therefore total possible outcomes are 2400

(i) The number of families earning Rs 10000 -13000 per month and owning exactly 2 vehicles are = 29, therefore favourable outcomes are = 29

P( 2 vehicle with income Rs 10000-Rs 13000) = 29/2400

(ii) The number of families earning Rs 16000 or more per month and owning exactly 1 vehicle are = 579, therefore favourable outcomes are = 579

P( 1 vehicle with income Rs 16000 or more) = 579/2400

(iii) The number of families earning less than ₹ 7000 per per month and doesn’t own any vehicle are = 10, therefore favourable outcomes are = 10

P( doesn’t own any vehicle with less than ₹ 7000) = 10/2400 = 1/240

(iv) The number of families earning ₹13000-16000 per month and owning more than 2 vehicles are = 25, therefore favourable outcomes are = 25

P(more than 2 vehicle with earning ₹13000-16000) = 25/2400 = 1/96

(v) The number of families owning not more than 1 vehicle are = Number of vehicles which has 0 vehicles + Number of families which has 1 vehicles = (10 + 1+2+1 ) +(160+305+535+479+579) = 14 +2048 =2062,therefore favourable outcomes are = 2062

P(family which has not more than 1 vehicle) = 2062/1400 =1031/1200

Q6.A teacher wanted to analyse the performance of two sections of students in a mathematics test of 100 marks. Looking at their performances, she found that a few students got under 20 marks and a few got 70 marks or above. So she decided to group them into intervals of varying sizes as follows
0 – 20, 20 – 30, …, 60 – 70, 70 – 100. Then she formed the following table

(i) Find the probability that a student obtained less than 20% in the mathematics test.
(ii) Find the probability that a student obtained marks 60 or above.

Ans.The number of students in both sections are = 90, therefore total possible outcomes are 90

(i) The number of students who obtained less than 20% marks are = 7,therefore favourable outcomes are 7

P(students who obtained less than 20% marks) = 7/90

(ii) The number of students who obtained marks 60 or above = marks in 60-70 + marks above 70 = 15 +8 = 23,therefore favourable outcomes are 23

P(students who obtained marks 60 or above) = 23/90

Q7.To know the opinion of the students about the subject statistics, a survey of 200 students was conducted. The data is recorded in the following table

Find the probability that a student is chosen at random
(i) likes statistics,
(ii) does not like it.

Opinion

Number of Students

Like

Dislike

135

Ans. Total number of students surveyed = 200,hence total possible outcomes are 200

(i) The number of students who likes statistics are 135,therefore favourable outcomes are 135

∴ P(students like statistics) = 135/200 = 27/40

Therefore the probabilty of the students who like statistics is 27/40

(ii) The number of students who dislike statistics are 65,therefore favourable outcomes are 65

∴ P(students like statistics) = 65/200 = 13/40

Therefore the probabilty of the students who dislike statistics is 13/40

Q8.The distance (in km) of 40 engineers from their residence to their place of work were found as follows

5	3	10	20	25	11	13	7	12	31
19	10	12	17	18	11	32	17	16	2
7	9	7	8	3	5	12	15	18	3
12	14	2	9	6	15	15	7	6	12

What is the empirical probability that an engineer lives
(i) less than 7 km from her place of work?
(ii) more than or equal to 7 km from her place of work?
(iii) within 1/2 km from her place of work?

Ans.The number of engineers whose distance of residence from their work place is highlighted here are 40,hence total possible outcomes are 40

(i) The distances lesss than 7 km are 5 km,3 km,2 km,3 km,5 km,3 km,2 km,6km and 6 km,hence number of engineers whose distance from their work place is less than 7 km are = 9 therefore favourable outcomes are 9

P(engineers whose residence is at less than 7 km) = 9/40

(ii) The distances more than or equal to 7 km are 10 km,20 km,25 km,11 km,13 km,7 km,12 km,31,km , 19 km,10 km,12 km,17 km,18 km,11 km,32 km,17 km,16 km,7 km,9 km,7 km,8 km,12 km,15 km,18 km,12km,14 km,9 km,15 km,15 km,7 km,12 km,hence number of engineers whose distance from their work place is more than or equal to 7 km are = 31 therefore favourable outcomes are 31

∴P(engineers whose residence is at more than or equal to 7 km) = 31/40

(iii) The number of engineers whose residence lies within 1/2 km from her place of work are =0.hence favourable outcomes are 0

∴ P(residence within 1/2 km from her place of work) = 0/40 = 0

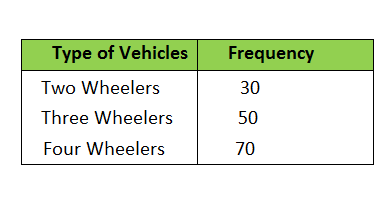
Q9.Activity: Note the frequency of two-wheelers, three-wheelers and four-wheelers going past during a time interval, in front of your school gate. Find the probability that any one vehicle out of the total vehicles you have observed is a two-wheeler?

Ans. Let the vehicles passing in front of a school gate after every one hour are listed below.

Ans. Total number of vehicles passing after every one hour are = 30 +50 +70 = 150,therefore total possible outcomes are 150

The number of two wheelers passing are =30

∴ P( two-wheelers passed) = 30/150 = 1/5

Hence the probability of observing that passed vehicle is two wheelers among all the vehicles is 1/5.

Q10.Activity : Ask all the students in your class to write a 3-digit number. Choose any student from the room at random. What is the probability that the number written by her/him is divisible by 3? Remember that a number is divisible by 3, if the sum of its digit is divisible by 3.

Ans. Let the number of students in the class are 20 and they have written a three digit numbers as listed below,therefore total possible outcomes are 20

121,123,230,990,674,253,133,450,555,897,784,846,987,340,983,675,765,245,354,405

The numbers which are divisible by 3 are 123,990,450,555,897,846,675,765,354,405,hence favourable outcomes are 10

P(number written by her/him is divisible by 3) = 10/20 = 1/2

Therefore probability that the number written by her/him is divisible by 3 is 1/2

Q11.Eleven bags of wheat flour, each marked 5 kg, actually contained the following weights of flour (in kg)
4.97, 5.05, 5.08, 5.03, 5.00, 5.06, 5.08, 4.98, 5.04, 5.07, 5.00
Find the probability that any of these bags,chosen at random contains more than 5 kg of flour.

Ans. The given bags of wheat flour each marked 5 kg weight of flour (in kg) are

4.97, 5.05, 5.08, 5.03, 5.00, 5.06, 5.08, 4.98, 5.04, 5.07, 5.00,therefore total possible outcomes are 11

The number of bags whose weight of flour (in kg) more than 5 kg are

5.05, 5.08, 5.03, 5.06, 5.08, 5.04, 5.07,therefore favourable outcomes are 7

P(weight of flour more than 5 kg ) = 7/11

Hence the probability of bags, chosen at random contains more than 5 kg of flour is 7/11

Q12.A study was conducted to find out the concentration of sulphur dioxide in the air in parts per million (ppm) of a certain city. The data obtained for 30 days is as follows

.0 3	0.08	0.08	0.09	0.04	0.17
.16	0.05	0.02	0.06	0.18	0.20
.11	0.08	0.12	0.13	0.22	0.07
.08	0.01	0.10	0.06	0.09	0.18
0.11	0.07	0.05	0.07	0.01	0.04

You were asked to prepare a frequency distribution table, regarding the concentration of sulphur dioxide in the air in parts per million of a certain city for 30 days. Using this table, find the probability of the concentration of sulphur dioxide in the interval 0.12-0.16 on any of these days.

Ans.The data obtained for 30 days of concentration of sulphur dioxide,therefore total possible outcomes are 30

The number of days in which concentration of sulphur dioxide in the interval 0.12-0.16 are 0.12,0.13,,hence favourable outcomes are 2

P(concentration of sulphur dioxide in the interval 0.12-0.16) = 2/30 = 1/15

Therefore the probability of the concentration of sulphur dioxide in the interval 0.12-0.16 is 1/15

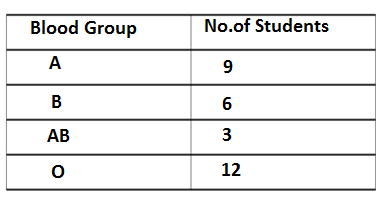
Q13. The blood groups of 30 students of class VIII are recorded as follows

A, B, O, O, AB, O, A, O, B, A, O, B, A, O, O, A, AB, O, A, A, O, O, AB, B, A, O, B, A, B, O

You were asked to prepare a frequency distribution table regarding the blood groups of 30 students of a class. Use this table to determine the probability that a student of this class, selected at random, has blood group AB.

Ans. The frequency distribution table of the blood groups of 30 students of a class is as follows.

The total number of students who are surveyed to check their blood group is 30,therefore total possible outcomes are = 30

The number of students who are found to have the blood group AB is 3,therefore favourable outcomes are = 3

P(the students have blood group AB) = 3/30 = 1/10

Hence the probability of the students have blood group AB is 1/10

NCERT Solutions of class 9 science

Chapter 1-Matter in our surroundings	Chapter 9- Force and laws of motion
Chapter 2-Is matter around us pure?	Chapter 10- Gravitation
Chapter3- Atoms and Molecules	Chapter 11- Work and Energy
Chapter 4-Structure of the Atom	Chapter 12- Sound
Chapter 5-Fundamental unit of life	Chapter 13-Why do we fall ill ?
Chapter 6- Tissues	Chapter 14- Natural Resources
Chapter 7- Diversity in living organism	Chapter 15-Improvement in food resources
Chapter 8- Motion	Last years question papers & sample papers

CBSE Class 9-Question paper of science 2020 with solutions

CBSE Class 9-Sample paper of science

CBSE Class 9-Unsolved question paper of science 2019

NCERT Solutions of class 10 maths

Chapter 1-Real number	Chapter 9-Some application of Trigonometry
Chapter 2-Polynomial	Chapter 10-Circles
Chapter 3-Linear equations	Chapter 11- Construction
Chapter 4- Quadratic equations	Chapter 12-Area related to circle
Chapter 5-Arithmetic Progression	Chapter 13-Surface areas and Volume
Chapter 6-Triangle	Chapter 14-Statistics
Chapter 7- Co-ordinate geometry	Chapter 15-Probability
Chapter 8-Trigonometry

CBSE Class 10-Question paper of maths 2021 with solutions

CBSE Class 10-Half yearly question paper of maths 2020 with solutions

CBSE Class 10 -Question paper of maths 2020 with solutions

CBSE Class 10-Question paper of maths 2019 with solutions

NCERT solutions of class 10 science

Chapter 1- Chemical reactions and equations	Chapter 9- Heredity and Evolution
Chapter 2- Acid, Base and Salt	Chapter 10- Light reflection and refraction
Chapter 3- Metals and Non-Metals	Chapter 11- Human eye and colorful world
Chapter 4- Carbon and its Compounds	Chapter 12- Electricity
Chapter 5-Periodic classification of elements	Chapter 13-Magnetic effect of electric current
Chapter 6- Life Process	Chapter 14-Sources of Energy
Chapter 7-Control and Coordination	Chapter 15-Environment
Chapter 8- How do organisms reproduce?	Chapter 16-Management of Natural Resources

Solutions of class 10 last years Science question papers

CBSE Class 10 – Question paper of science 2020 with solutions

CBSE class 10 -Latest sample paper of science

NCERT solutions of class 11 maths

Chapter 1-Sets	Chapter 9-Sequences and Series
Chapter 2- Relations and functions	Chapter 10- Straight Lines
Chapter 3- Trigonometry	Chapter 11-Conic Sections
Chapter 4-Principle of mathematical induction	Chapter 12-Introduction to three Dimensional Geometry
Chapter 5-Complex numbers	Chapter 13- Limits and Derivatives
Chapter 6- Linear Inequalities	Chapter 14-Mathematical Reasoning
Chapter 7- Permutations and Combinations	Chapter 15- Statistics
Chapter 8- Binomial Theorem	Chapter 16- Probability

CBSE Class 11-Question paper of maths 2015

CBSE Class 11 – Second unit test of maths 2021 with solutions

NCERT solutions of class 12 maths

Chapter 1-Relations and Functions	Chapter 9-Differential Equations
Chapter 2-Inverse Trigonometric Functions	Chapter 10-Vector Algebra
Chapter 3-Matrices	Chapter 11 – Three Dimensional Geometry
Chapter 4-Determinants	Chapter 12-Linear Programming
Chapter 5- Continuity and Differentiability	Chapter 13-Probability
Chapter 6- Application of Derivation	CBSE Class 12- Question paper of maths 2021 with solutions
Chapter 7- Integrals
Chapter 8-Application of Integrals