Solutions of class 12  maths question paper 2021 preboard exam CBSE

Solutions of class 12 CBSE maths question paper 2021 preboard exam are created for helping the students of 12 class in CBSE board exam. The solutions of the question paper will give a complete idea about the type of questions and the ways of solving them. All solutions of class 12 CBSE maths question paper 2021 preboard exams are solved by an expert of maths subject. We hope these solutions will definitely help to boost the preparation of maths question papers in the board exam of CBSE.

This question paper contains two parts-A and B. Each part is compulsory. Part A carries 24 marks and part B has descriptive-type questions of 56 marks.

Part A

Section I -16 questions(1 marks each)

Section II -2 case studies. Each case study comprises of 5 case-based MCQ’s.questions (1 marks each)

Part B

Section III-10 questions of 2 marks each

Section IV-07 questions of 3 marks each

Section V-03 questions of 5 marks each

Solutions of class 12  maths question paper 2021 preboard exam CBSE

Part -A

Section-I

Q1. Determine whether relation R in the set N of natural number defined as:

R = {(x,y) : y = x + 5 and x < 4} is symmetric.

Ans. The relation given us in the the set N of natural number

R = {(x,y) : y = x + 5 and x < 4}

x < 4 implies that x= 1,2,3

For x = 1,y = 1 +5 =6,for x = 2,y = 2+ 5 =7, for x =3,y = 3 +5=8

Therefore

R = {(1,6),(2,7),(3,8)}

Since (1,6) ∈ R but (6,1) ∉ R,so R is not symmetric

Q2.Let A = R – {3}, B = R – {1},consider the function f : A →B defined by

f(x) = (x -2)/(x -3). Check whether function is one-one or not.

Ans. We are given: A = R – {3}

B = R – {1}

F: A→B defied as

(x₁ ∉ 3)

If f(x₁) ₌ f(x₂) then

x₁= x₂

Tis implies that x₁= x₂ therefore is on-on

Q3.Let Set A = {1,2,3} define the relation R in the set A as follows:R ={(1,1),(2,2),(3,3),(1,3)} which order to be added to R make it smallest equivalence relation.

Ans. A relation is said to be an equivalence relation when it is transitive,reflexive and symmetric

For the  Set A = {1,2,3}, R={(1,1),(2,2),(3,3),(1,3)}

For the Set A the relation is reflexive a ∈A, (a,a) ∈ R ,so minimum order  added should be (1,1),(2,2),(3,3)

For the Set A the relation is symmetric , a ∈A,b∈A, (a,b) ∈ R and (b,a) ∈R ,so minimum order added should be {(1,3),(3,1}

For the Set A the relation is transitive , (a,b) ∈A,(b,c) ∈A then (a,c) ∈ R ,the order must be  {(1,3),(3,3)}∈R,so (1,3) ∈ R

Therefore order to be added to R make it smallest equivalence relation is (3,1)

OR

Show that function f from z →z defined as f(x) = x + 2 is bijective.

Ans. For every value of x ∈z, f(x) = x +2: f(1) = 1+2 =3,f(2) =2+2=4 ,f(1) ≠f(2) for 1 ≠ 2, for every x ∈z,there is distintive image of z in z,so given function is one to one relation

For every value of x ∈ z,there is atleast one image of f in z: f(1) =2, 2∈z,f(o) =2 it indicates that for f(1) = f(2) ,0≠1,so function is onto relation

Since the function is one to one as well as onto, therefore it is bijective

Q4. Find the number of all possible matrices of order 3 ×3 with each entry 0 or 1.

Ans. The number of all possible entries in the matrix of order 3 ×3 =9

The number of choices to fill every entry is,2 = 0,9

So, the number of total possible   matrices are =2⁹ =512

Ans. We are given A, B and C matrix, such that 2A + 3B – 2C ia a null matrix where

 

According to question

OR

Q7.Find ∫ e^x sec x(1+tanx)dx

Ans.∫ e^x secx(1+tanx)dx

= ∫ e^x (secx + secx.tanx)dx

It is of the form

∫ e^x[f(x) + f′(x)]dx = e^x f(x) + C

where f(x) = secx

f′(x) = secx.tanx

so, our equations becomes

∫ e^x (secx + secx.tanx)dx = e^x secx + C

OR

Ans. We are given

Using the integral formula

2a = 1

a = 1/2

Solutions of class 12  maths question paper 2021 preboard exam CBSE

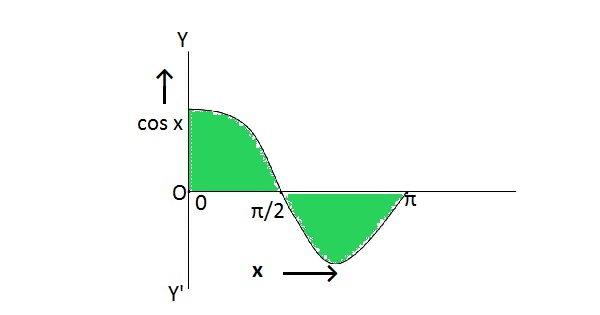
Q8. Find the area of region bounded by curve y = cos x between x = 0 and x = π.

Ans. The graph of the curve  y = cos x  between x = 0 and x = π is as shown below

The graph y = cosx is a periodic function,so the area from x =0 to x =π/2 = area from π/2 to π is same.

Area from x= 0 to π = 2× area of graph from 0 to π/2

Area from x= 0 to π = 2×Integration of cos x within the limit 0 to π/2

= 2(sinπ/2 – sin0)

= 2

Therefore area of region bounded by curve is 2 sq.unit

Q9. Find the degree of differential equation.

Ans. Highest order of derivative is (d²x/dx²) and its degree is 2

Hence the degree of differential equation is 2

OR

Find the integrating factor of differential equation

Ans. The differential equation  given to us is

The integration factor (I) of linear equation dy/dx +py = Q is given as following

Rearranging the  given equation in the standard form

dy/dx -y/x = 2x²

On comparing the standard equation and given equation ,we have p = -1/x

I = 1/x ( Since e^logx = x)

Please follow us on pintrest

Pintrest future study poin

Q10. Find the direction ratio of vector    and hence calculate its direction cosines.

Ans. The given vector is = i +j -2k, its direction ratios are a =1,b = 1 and c = -2

The magnitude of = i +j -2k is = = √[1²+1²+(-2)²] =√(1 +1 +4) =√6

The direction cosines of vector are

Q11. Find the area of the parallelogram whose adjacent sides are determined by vectors. 

Ans. Area of the parallelogram whose adjacent sides are (i-j +3k) and (2i -7j +k) is given by =

= [-1 – (-21) ]i – [1-6]j + [-7-(-2)]k

= 20i + 5j -5k

Area of the parallelogram is = 15√2 square unit.

Q12.If is a unit vector and   

Ans. We are given is a unit vector such that

Q13.Find the equation of the line which is parallel to      and passes through the point (5,2,-4).

Ans. Equation of the line passing throgh (5,2,-4) is

The given line is

Equation of a line passing through a point and parallel to another vector is given as

, where is the position vector of the required line and λ is a scaler

Solutions of class 12  maths question paper 2021 preboard exam CBSE

Q14. Find the distance between two planes:2x +3y +4z=4 and 4x +6y +8z =12

Ans. The distance between two planes Ax +By +Cz = d₁ and Ax +By +Cz = d₂

is given by

The given planes are 2x +3y +4z=4 and 4x +6y +8z =12,rearranging the given second plains =2(2x+3y +4z)=12⇒2x +3y +4z=6

On comparing the equations of given plains with the equations standard plains, we have

A =2, B =3, C = 4, d₁= 4, d₂=6, Let D is the distance between two plains

Q15. Evaluate P(A∪B) if 2P(A) = P(B) = 5/13 and P(A/B) = 2/5.

Ans. Given, 2P(A) = P(B) = 5/13

So, P(A) = 1/2 × 5/13 = 5/26

And P(B) = 5/13

Also, P(A|B) = 2/5

We know, P(A|B)= P(A B)/P(B)

Also, P(A B) = P(A) + P(B) – P(A B)

Q16. Event A and B are such that P(A) =1/2, P(B) =7/12, and P(not A nor B)=1/4. State whether A and B are independent?

Ans. It is given that P(A) = 1/2, P(B) = 7/12, and P(not A nor B)=1/4

P(A′ B′) = 1/4

However, P(A) – P(B) = 1/2 × 7/12 = 7/24…(2)

Here, 2/4 ≠ 7/24

∴ P(A B) ≠ P(A).P(B)

Therefore, A and B are not independent events.

SECTION-II

Both case study-based questions are compulsory. Attempt any 4 sub-parts of each question 17 and 18. Each question carries 1 mark.

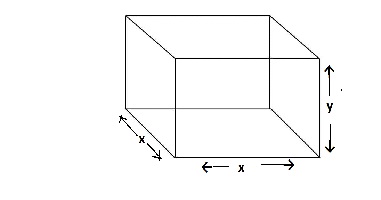
Q17.In a box manufacturing company during practical ability test of B.Tech for the post of an engineer,instructor of company instructed a participant to construct an open box with square base with a given quantity of cardboard of area C².

(i) If length of side of square base be x units and height is y units .Then area of material used is:

(a) x² + 4xy              (b) x + 4x²y

(c) x + 4xy²             (d) y² +4xy

Ans. Since the box is open,therefore the  area of cardboard required

2×height(length + breadth)

2× y(x +x) + x×x

x² +4xy

Hence answer is (a)

(ii) The relation between x and y is :

(a) x = (c² -x²)/4y    (b) y =(c²-x²)/4x

(c) x = (x²-c²)/4y     (d) y =(x²-c²)/4x

Ans. Area of the cardboard = area of the box =c²

Area of the open box = x² +4xy

x² +4xy = c²⇒y = (c²-x²)/4x

Hence answer is (ii)

(iii) Volume of box expressed as function of x is :

(a) 1/4(c² x -x³)       (b) 1/4(x³- c²x)

(c) 1/4(x²- cx)         (d) 1/4(cx – x²)

Ans. Volume of the box,V = l×b×h = x × x×y= x²y

As it is calculated above in (i) y = (c²-x²)/4x

V = x²(c²-x²)/4x = x(c²-x²)/4= 1/4(c² x -x³)

Hence answer is (i)

(iv) At what value of ‘x’ volume is maximum ?

(a) x = c/3               (b) x = c²/√3

(c) x = c/√3            (d) x =√3 c

Ans.

For getting maximum value of volume ,the slope of the V-x curve should be 0.

V = 1/4(c²x – x³)

dV/dx = 1/4 d/dx(c²x – x³) = 1/4(c² – 3x²)

1/4(c² – 3x²) = 0

c² – 3x² = 0

x = c/√3

Hence answer is (iii)

(v) Maximum value of V is :

(a) c²/6√3            (b) c/3√6

(c) c²/6√3           (d) c/6√3

Ans. As we have determined in (iv) the value of x for which volume is maximum

So putting the value of x = c/√3 in volume equation

V = 1/4(c²x – x³)

V(max.) = 1/4[c² ×c/√3 – (c/√3)³]

= 1/4[c³/√3 -c³/3√3]

= c³/4√3[1-1/3] = 2c³/12√3 = c³/6√3

Hence answer is (iii)

Solutions of class 12  maths question paper 2021 preboard exam CBSE

Q18. A car manufacturing factory has two plants X and Y.Plant X manufactures 70 % of cars and plant Y manufactures 30 %. 80% of cars at plant X and 90 % of cars at plant Y are rated of standard quality. A car is chosen at random and is found to be of standard quality.

Let A = Event car of standard quality

B₁  =Event car is manufactured in X

B₂ = Event car is manufactured in Y

Based on the information given above answer the following (any four)

(i) Value of P(B₁ ) will be:

(a) 70 %                 (b) 7/10

(c) 70/10               (d) 3/10

Ans.B₁  =Event car is manufactured in X

P(B₁) →Probability of the car that is manufactured in X plant

P(B₁) = 70 %

Hence answer is (a)

(ii) Value of P(B₂ ) will be:

(a) 10/3                (b) 30%

(c) 3/10               (d) 7/10

B₂  =Event car is manufactured in Y

P(B₂) →Probability of the car that is manufactured in Y plant

P(B₂) = 30 %

Hence answer is (b)

(iii) Probability that standard quality car is manufactured in plant X will be:

(a) 9/10               (b) 8/10

(c) 7/10               (d) 3/10

Ans. The probability that a standard quality car is manufactured in plant X

It means if the selected car is  manufactured in plant X then it is of standard quality , its probability is given by = P(E/B₁) =80% =  8/10

Hence answer is (ii)

(iv) Probability that standard quality car is manufactured in plant Y will be:

(a) 9/10               (b) 8/10

(c) 7/10               (d) 3/10

Ans. The probability that a standard quality car is manufactured in the plant Y

It means if the selected car is  manufactured in plant Y then it is of standard quality, its probability is given by = P(E/B₂) =90% =  9/10

(v) If a car is selected at random is of standard quality. What is the probability standard quality car has manufactured from plant X.

(a) 83/56              (b) 56/100

(c) 56/83               (d) 27/100

Ans. The probability that a standard quality car is manufactured in plant X

It means if the selected car is of standard quality then it is manufactured in plant X ,its probability is given by = P(B₁/E)

Applying Bays theorem

Where P(B₁) = 70 %  =0.70, P(B₂) = 30 %=0.30

P(E/B₁) = 80% =0.80, P(E/B₂) = 90%=0.90

Part -B

SECTION-III

Q19. Write           in the simplest form.

Ans. We are given the expression

Let x = secθ, θ = Sec^-1x

Q20.Find the value of x, y, and z if the matrix

satisfying equation AA´= I

Ans. The matrix(A) given to us

A’ → Transpose of the matrix A

I→ Unit matrix of the order 3× 3

According to question

AA’ = I

Since matrices are equal so corresponding elements will be equal

2x² =1⇒ x =±1/√2, 6y² = 1⇒y =±1/√6,3z²=1⇒z=±1/√3

OR

Find the non-zero values of x satisfying, Matrix equation:

Ans.The matrix equation is given to us

On comparing the corresponding elements

3x +8 = 20 ⇒ x = 4, this value of x also satisfy other equations 2x+12x = 48,x² +8x = 12x

Q21.Find the relationship between a and b. So that function ‘f’ defined.

is continuous at x = 3

Ans. As we know a function f(x) is continuous at x= a, when its left-hand limit , right-hand limit at x =a is equal to the value of the function at x =a.

Left-hand limit of the function( the function  defined  for x<3 is f(x) = ax +1)

Right-hand limit of the function( the function  defined  for x>3 is f(x) = bx +3)

Since it is given to us that function is continuous at x =3

∴ LHL = RHL

3a + 1 = 3b +3

3a – 3b =2

Therefore required relation is 3a – 3b =2

 

Q22.Find the equations of all lines having slope zero which are tangent to the curve     

Ans. We are given the curve

We are given,the slope of the curve is 0, so dy/dx = 0

x = 1

Value of y when x = 1

The slope is passing through the coordinates (1,1/2)

The equations of the lines through the point (x₁,y₁) is given as

(y-y₁) = m(x-x₁)

(y-1/2) = 0(x -1)

y-1/2 = 0

Therefore the equation of the tangent is y-1/2 = 0

Solutions of class 12  maths question paper 2021 preboard exam CBSE

Q23.Integrate: ∫ cos³ x e^{log sinx} dx

Ans. The integral function given to us is

∫ cos³ x e^{log sinx} dx

I = ∫ cos³ x sinx dx   [e^{log x} = x]

Let t = sinx

dt/dx = cos x

Putting the value of dt and t in the function

Putting the value sinx =t

Substituting the value of t= sin x

Therefore the integration of the given function is

OR

Ans. The given function is

f(x)=x¹⁷.cos⁴ x

Putting the value x = -x

f(-x) =(-x)¹⁷.cos⁴ (-x)

f(-x) = -x¹⁷.cos⁴ x = -f(x)

∴ f(x) is an odd function

Using the property

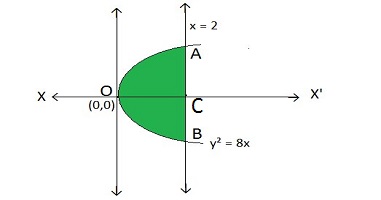
Q24. Find the area of the region bounded by line x = 2 and parabola y² = 8x.

Ans. The equations of parabola y² = 8x and of the line  is x = 2

Drawing the graph of the parabola and the line

The area bounded by the parabola and the line is = 2× area of AOC

Area of AOC = Integration of the function y with respect to x within the limits x =0 and x =2.

y² = 8x

y  =±2√2√x

Since y is in the first quadrant

∴ y =  2√2√x

The area bounded by the parabola and the line is (AOBC)

Q25.For given differential equations find the particular solutions satisfying giving condition:

Ans. The differential equation given is

y = c sec x

Putting values of y =1, x = 0

1 =c sec 0⇒ c = 1`

Putting value of c = 1,we get

y = 1 ×sec x

y = sec x

Therefore required particular solution of the given differential equation is y= sec x.

Q26. If    such that

  is perpendicular to  Find the value of λ.

Ans. We are given that

  is perpendicular to

Since    is perpendicular to

(2-λ)3 + (2 + 2λ) = 0

6- 3λ + 2 + 2λ = 0

8 – λ = 0

λ = 8

Therefore the required value of λ is = 8

Solutions of class 12  maths question paper 2021 preboard exam CBSE

Q27. Show that line joining the origin to the point (2,1,1) is perpendicular to the line determined by points (3,5,-1) and (4,3,-1).

Ans. Let the origin is O(0,0,0) and the point A(2,1,1) , the points of another line B(3,5,-1) and C(4,3,-1)

A line passing through (x_1, y_1, z₁) and (x_2, y_2, z₂) has the direction ratios

(x₂ – x₁), (y₂ – y₁), (z₂ – z₁)

Direction ratios of OA =(2-0),(1-0),(1-0)= 2,1,1

Direction ratios of BC =(4-3),(3-5),(-1+1)= 1,-2,0

Two lines having direction ratios a_1, b_1, c₁ and a_2, b_2, c₂ are perpendicular to each other if

a₁ a₂ + b₁ b₂ + c₁ c₂ = 0

a₁ = 2, b₁ = 1, c₁ = 1, a₂ = 1, b₂ = -2, c₂ = 0

2×1+1×-2+1×0 =2-2+0 = 0

Therefore given two lines OA and BC are perpendicular to each other

Q28. An urn contains 10 black and 5 white balls. Two balls are drawn from the urn one after the other without replacement. What is the probability that both drawn balls are black?

Ans.We are given that an urn contains ,10-black balls,5-white balls

Total balls in the urn are = 10+5=15 and number of black balls=10

The probability of first ball drawn is black

= (number of black ball)/(Total ball) = 10/15= 2/3

Since two balls are drawn from the urn one after the other without replacement

∴ The probability of second ball drawn is black if first ball drawn is black = (number of remaining black ball)/(Total remaining ball)= 9/14

According to multiplication rule of probability = (2/3)× (9/14) =3/7

The probability that both drawn balls are black is 3/7

OR

If P(A) = 2/5, P(B) = 3/10, P(A∩B) = 1/5. Find the value of P(A’/B’)

Ans. We have to find out P(A’/B’) =?

As we know, P(A’/B’) = P(A’∩B’)/P(B’)

Since P(A’∩B’) = P(A∪B)

P(A’/B’) = P(A∪B)/P(B’)…….(i)

P(B’) = 1- P(B) = 1 – 3/10 = 7/10

Let’s find out P(A∪B),for this applying the following formula

P(A∪B) = P(A) + P(B) – P(∩B)

From the question,we have P(A) = 2/5, P(B) = 3/10, P(A∩B) = 1/5

P(A∪B) = 2/5 + 3/10 – 1/5 = 5/10 =1/2

Putting the value of P(B’) and P(A∪B) in (i)

P(A’/B’) = (1/2)/(7/10)= 10/14 = 5/7

Solutions of class 12  maths question paper 2021 preboard exam CBSE

Part -B

SECTION-IV

Q29. Let A = {1,2,3……9} and R be the relation in A × A defined by (a,b)R(c,d) if a +d = b+c for (a,b),(c,d) in A ×A. Prove that R is equivalence relation.Also obtain equivalence class [2,5].

Ans. We are given that  A = {1,2,3……9}and R be the relation in A × A defined by (a,b)R(c,d) if a +d = b+c for (a,b),(c,d) in A ×A

R in A × A

(a,b)R(c,d) if   (a,b),(c,d) ∈ A ×A

a +d = b+c

Considering (a,b) R (a,b)

a +b = b+a which is true for every value of (a,b) ∈ R(A ×A)

Hence R is reflexive

(a,b)R(c,d) if   (a,b),(c,d) ∈ A ×A

a +d = b+c

Checking it for (c,d)R(a,b)

c + b = d +a, which is true for every value of (c,d) ,(a,b)∈ R(A ×A)

(c,d) R(a,b) is true

Hence R is symmetric

Let (a,b)R(c,d)and(c,d)R(e,f)
a+d = b+c and c+f = d+e
⇒ a+d = b+c and d+e = c+f
⇒ (a+d) – (d+e) = (b+c) – (c+f)
⇒ a-e = b-f
⇒ a+f = b+e
(a,b)R(e,f)
So,R is transitive
Hence, R is an equivalence relation

Equivalence class [2,5] in A = {1,2,3,4,5,6,7,8,9}

a +d = b+c ⇒ a -b = c-d

2 – 5 = -3⇒ a-b =c-d =-3 for every (a,b),(c,d) ∈ A [2,5]

∴ Forming the ordered pair (c,d) satisfying the condition d= c+3

(2,5) R (c,d)

(2,5) R (1,4),(2,5),(3,6),(4,7),(5,8),(6,9)

It is symmetric also,so the following relations also true

(1,4)R(2,5),(3,6)R(2,5),(4,7)R(2,5),(5,8)R(2,5),(6,9)R(2,5)

Therefore there 11 equivalent class of [2,5]

Q30. Differentiate the function:

w.r.t. ‘x’

Ans.

Taking logarithms of both sides

 

Now, differentiating both sides

Q31. Find dy/dx, if x^2/3 + y^2/3 = a ^2/3

Ans.

Differentiating w.r.t x

OR

If y = sin^-1x show that:

(1 – x²) d²y/dx² – x(dy/dx) = 0

Ans. We are given y = sin^-1x

Differentiating both sides

Squaring both sides

Differentiating both sides

Hence Proved

Solutions of class 12  maths question paper 2021 preboard exam CBSE

Q32. Prove that y = (4sinθ/2+cosθ)-θ is an increasing function of θ in [0, π/2]

Ans. The given function is

Differentiating both sides w.r.t θ

 

Observing right hand side cosθ > 0 for θ ∈[0,π/2],(4-cosθ)>0 since -1≤cosθ≤1

(2+cosθ)² is always + ve.

Hence y = (4sinθ/2+cosθ)-θ is an increasing function of θ in [0, π/2]

The integral function is given to us

Let  u = cos x

du = sin x.dx

Substituting the value u = cos x and du =sin x.dx

Substituting back the value u = cos x

= 1/3 -1 = -2/3

OR

Evaluate:

Ans. Evaluating the given integral function

t = x⁴ + 1

dt/dx = 4x³

dt = 4x³ dx ⇒ dx = dt/4x³

Substituting x⁴ + 1 = t and value of dx in the function

Since x⁴ + 1 is always positive

Q34. Find the area of the region bounded by y² = 9x. x = 2, x = 4 and x-axis in the first quadrant.

Ans.  The graph of the curve  y² = 9x and the lines x = 2, x = 4  is shown below.

We have to find out the area bounded by x -axis, y² = 9x, y = 2 and y = 4 which is ABCD

The area of ABCD = Integration of  y w.r.t x within the limits x =2 and x =4

y² = 9x ⇒ y = 3√x

= 16 -4√2

Hence area bounded by x -axis, y² = 9x, y = 2 and y = 4 is (16 -4√2) sq.unit

Q35. Find the general solution of the following differential equation

Cos²x (dy/dx) + y = tan x, 0 ≤ x ≤ π/2

Ans. The given equation is

Writing the given equation in the form of the general equation  dy/dx +Py = Q

Where P = sec²x and Q = tanx.sec²x

We know the general solution of the equation dy/dx +Py = Q

y ×(I.F) = ∫(Q × I.F) dx  +C

It is known to us that the integrating factor(I.F) is calculated as follows

I.F = e^∫Pdx=e^∫sec²dx =e^tanx

Putting the value of Q = tanx.sec²x and of I.F in the general solution

y e^tanx =∫(tanx.sec²x) e^tanx dx  +C

Let t = tanx ⇒ dt/dx = sec²x ⇒ dt = dx.sec²x

Substituting tanx = t and dx.sec²x = dt

ye^t=∫t.dt .e^t+ C

ye^t= t.e^t– e^t+ C

y = t -1 + C/e^t

Putting the value of t = tanx

y = tanx  + C’ is the required solution where -1+ C/e^t = C’

Solutions of class 12  maths question paper 2021 preboard exam CBSE

Part -B

SECTION-V

Q36. Use product:

to solve the system of equations:

3x – 2y + 3z = 8, 2x +y – z = 1, 4x – 3y + 2z = 4

OR

Solve the following system of equations by matrix method:

3x + 2y – 2z = 3

x + 2y + 3z = 6

2x – y + z = 2

Ans. Given system of equations is:

3x + 2y – 2z = 3

x + 2y + 3z = 6

and 2x – y + z = 2

or AX = B

X = A^-1 B

∴ For A^-1, Cofactors are

A₁₁ = 5, A₁₂= 5, A₁₃ = -5,

A₂₁ = 0, A₂₂= 7, A₂₃ = 7,

A₃₁ = 10, A₃₂= -11, A₃₃ = 4

|A| = 3(5) + 2(5) + (-2)(-5) = 35

 

∴ x = 1, y =1 and z = 1

Q37. Find the shortest distance between lines whose vector equations are:

Ans. The shortest distance between two lines   is

We are given here the vector equations

On comparing these equations with standard vector equations

We have

 

Therefore the shortest distance between both of the lines is

 

OR

Find the equation of the plane which contains the line of intersection of the planes and  which is perpendicular to the plane

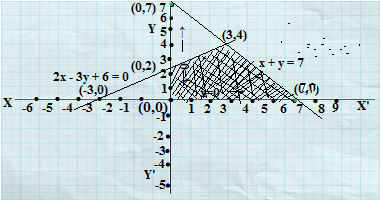
Q38.Minimize z = 13x – 15y

Such that x + y ≤ 7

2x – 3y + 6 ≥ 0, x ≥0, y ≥0

Ans. We have to minimize  z=13x – 15y such that x + y ≤ 7,2x – 3y + 6 ≥ 0, x ≥0, y ≥0

Solutions of the equation x + y = 7 , for x = 0,y = 7 and for y=0,x = 7,ploting the line between (0,7) and (7,0)

Solutions of the equation 2x – 3y + 6 = 0, for x = 0, y= 2, for y=0, x= -3,plotting the lines between (0,2) and (-3,0)

The solutions of the equation x = 0,is y axis and of y= 0 is x axis

Locating the co-ordinates (0,7),(7,0),(0,2),(-3,0) and (0,0)

 

 

The corner points of the graph of inequalities are (0,0),(3,4),(0,2),(7,0)

Evaluating z=13x – 15y on every points (0,0),(3,4),(0,2),(7,0)

On (0,0), z = 0, on (3,4) , z = 13×3 -15×4 =39-60 =-21, on (0,2), z =-30,on (7,0), z =91

The minimum value of z is -30

OR

The corner points of feasible region of LPP are (0,8), (4,10), (6,8), (6,5), (0,0) and (5,0). Let the objective function be z = 3x – 4y. Answer the following:

(a) Find the point at which the minimum value of z occurs.

(b) Find the point at which the maximum value of z occurs.

(c) Find the sum of the minimum value of z and the maximum value of z.

(d) Let z = px + qy, where p, q > 0

Ans.Find the relation between p and q, so that minimum of z occurs at (0,8) and (4,10).

You can compensate us by donating any amount of money for our survival

Our Paytm NO 9891436286

NCERT Solutions of Science and Maths for Class 9,10,11 and 12

NCERT Solutions for class 9 maths

Chapter 1- Number System	Chapter 9-Areas of parallelogram and triangles
Chapter 2-Polynomial	Chapter 10-Circles
Chapter 3- Coordinate Geometry	Chapter 11-Construction
Chapter 4- Linear equations in two variables	Chapter 12-Heron’s Formula
Chapter 5- Introduction to Euclid’s Geometry	Chapter 13-Surface Areas and Volumes
Chapter 6-Lines and Angles	Chapter 14-Statistics
Chapter 7-Triangles	Chapter 15-Probability
Chapter 8- Quadrilateral

NCERT Solutions for class 9 science 

Chapter 1-Matter in our surroundings	Chapter 9- Force and laws of motion
Chapter 2-Is matter around us pure?	Chapter 10- Gravitation
Chapter3- Atoms and Molecules	Chapter 11- Work and Energy
Chapter 4-Structure of the Atom	Chapter 12- Sound
Chapter 5-Fundamental unit of life	Chapter 13-Why do we fall ill ?
Chapter 6- Tissues	Chapter 14- Natural Resources
Chapter 7- Diversity in living organism	Chapter 15-Improvement in food resources
Chapter 8- Motion	Last years question papers & sample papers

NCERT Solutions for class 10 maths

Chapter 1-Real number	Chapter 9-Some application of Trigonometry
Chapter 2-Polynomial	Chapter 10-Circles
Chapter 3-Linear equations	Chapter 11- Construction
Chapter 4- Quadratic equations	Chapter 12-Area related to circle
Chapter 5-Arithmetic Progression	Chapter 13-Surface areas and Volume
Chapter 6-Triangle	Chapter 14-Statistics
Chapter 7- Co-ordinate geometry	Chapter 15-Probability
Chapter 8-Trigonometry

CBSE Class 10-Question paper of maths 2021 with solutions

CBSE Class 10-Half yearly question paper of maths 2020 with solutions

CBSE Class 10 -Question paper of maths 2020 with solutions

CBSE Class 10-Question paper of maths 2019 with solutions

NCERT Solutions for Class 10 Science

Chapter 1- Chemical reactions and equations	Chapter 9- Heredity and Evolution
Chapter 2- Acid, Base and Salt	Chapter 10- Light reflection and refraction
Chapter 3- Metals and Non-Metals	Chapter 11- Human eye and colorful world
Chapter 4- Carbon and its Compounds	Chapter 12- Electricity
Chapter 5-Periodic classification of elements	Chapter 13-Magnetic effect of electric current
Chapter 6- Life Process	Chapter 14-Sources of Energy
Chapter 7-Control and Coordination	Chapter 15-Environment
Chapter 8- How do organisms reproduce?	Chapter 16-Management of Natural Resources

NCERT Solutions for class 11 maths

Chapter 1-Sets	Chapter 9-Sequences and Series
Chapter 2- Relations and functions	Chapter 10- Straight Lines
Chapter 3- Trigonometry	Chapter 11-Conic Sections
Chapter 4-Principle of mathematical induction	Chapter 12-Introduction to three Dimensional Geometry
Chapter 5-Complex numbers	Chapter 13- Limits and Derivatives
Chapter 6- Linear Inequalities	Chapter 14-Mathematical Reasoning
Chapter 7- Permutations and Combinations	Chapter 15- Statistics
Chapter 8- Binomial Theorem	Chapter 16- Probability

CBSE Class 11-Question paper of maths 2015

CBSE Class 11 – Second unit test of maths 2021 with solutions

NCERT solutions for class 12 maths

Chapter 1-Relations and Functions	Chapter 9-Differential Equations
Chapter 2-Inverse Trigonometric Functions	Chapter 10-Vector Algebra
Chapter 3-Matrices	Chapter 11 – Three Dimensional Geometry
Chapter 4-Determinants	Chapter 12-Linear Programming
Chapter 5- Continuity and Differentiability	Chapter 13-Probability
Chapter 6- Application of Derivation	CBSE Class 12- Question paper of maths 2021 with solutions
Chapter 7- Integrals
Chapter 8-Application of Integrals

Class 12 Solutions of Maths Latest Sample Paper Published by CBSE for 2021-22 Term 2

Class 12 Maths Important Questions-Application of Integrals

Class 12 Maths Important questions on Chapter 7 Integral with Solutions for term 2 CBSE Board 2021-22

Solutions of Class 12 Maths Question Paper of Preboard -2 Exam Term-2 CBSE Board 2021-22

Solutions of class 12  maths question paper 2021 preboard exam CBSE Solution