NCERT Solutions for Class 9 Maths exercise 12.2 Chapter 12 Heron’s Formula

NCERT Solutions for Class 9 Maths exercise 12.2 Chapter 12 Heron’s Formula are solved here for helping the 9 class students in doing their homework and preparation of the class test, CBSE exams, and completing assignments of the chapter 12 Heron’s Formula. Heron’s formula is related to the area of triangles when all sides are given. The NCERT solutions of the exercise 12.2 chapter 12 Heron’s Formula will help you in clearing doubts on unsolved questions of the class 9 maths NCERT textbook.
Area of Δ = √[s(s -a)(s-b)(s-c)]
Where s is semiperimeter and a,b and c are the sides of the triangle
s = (a + b+ c)/2
Click for online shopping
Future Study Point.Deal: Cloths, Laptops, Computers, Mobiles, Shoes etc
NCERT Solutions for Class 9 Maths Chapter 12 Heron’s Formula
Exercise 12.1- Heron’s Formula
Exercise 12.2- Heron’s Formula
NCERT Solutions of class 9 maths
| Chapter 1- Number System | Chapter 9-Areas of parallelogram and triangles |
| Chapter 2-Polynomial | Chapter 10-Circles |
| Chapter 3- Coordinate Geometry | Chapter 11-Construction |
| Chapter 4- Linear equations in two variables | Chapter 12-Heron’s Formula |
| Chapter 5- Introduction to Euclid’s Geometry | Chapter 13-Surface Areas and Volumes |
| Chapter 6-Lines and Angles | Chapter 14-Statistics |
| Chapter 7-Triangles | Chapter 15-Probability |
| Chapter 8- Quadrilateral |
NCERT Solutions for Class 9 Maths exercise 12.2 Chapter 12 Heron’s Formula
Q1. A park in the shape of a quadrilateral ABCD has ∠C = 90°, AB = 9 m, BC = 12 m,CD = 5 m and AD = 8 m. How much area does it occupy.
Ans. We are given a quadrilateral ABCD , in which ∠C = 90°, AB = 9 m, BC = 12 m,CD = 5 m and AD = 8 m.
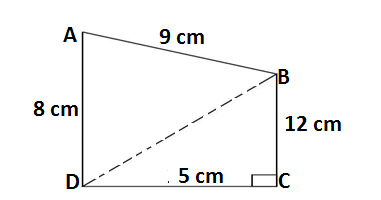
Since ΔBCD is a right triangle therefore applying the formula of area of triangle
ar Δ = 1/2(Base) ×Altitude
arΔ BCD = 1/2(DC) × (BC) = 1/2(5)(12) = 30 cm²
Determining the length of BD by applying the Pythagoras formula
BD = √(12² + 5²) = 13 cm
Now let’s find the area of the ΔABD by applying the Heron’s formula
a = 8 cm, b = 9 cm, c =13 cm
s = (a +b+c)/2 = (8 +9+13)/2 =15 cm
= 6√35 cm² =6 ×5.92 ≈35.5
Therefore area of ABCD = Area of BCD + Area of ABD = 30 +35.5 = 65.5 cm²
NCERT Solutions for Class 9 Maths exercise 12.2 Chapter 12 Heron’s Formula
Q2.Find the area of quadrilateral ABCD in which AB = 3 cm, BC = 4 cm, CD = 4 cm, DA = 5 cm and AC = 5 cm.
Ans. We are given a quadrilateral ABCD in which AB = 3 cm, BC = 4 cm,CD = 4 cm and AD = 5 cm and AC = 5 cm
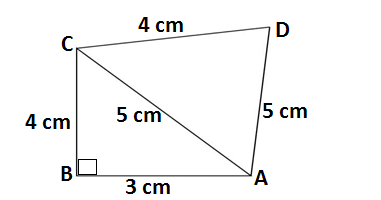
In ΔABC, AB = 3 cm, BC = 4 cm and AC = 5 cm
AC² = BC ² + AB² ⇒ 5² = 4² + 3²
Therefore ΔABC is a right triangle
Since ΔABC is a right triangle therefore applying the formula of area of triangle
ar Δ = 1/2(Base) ×Altitude
arΔ BCD = 1/2(AB) × (BC) = 1/2(3)(4) = 6 cm²
In ΔADC,we have CD = 4 cm, DA = 5 cm and AC = 5 cm.
Now let’s find the area of the ΔADC by applying the Heron’s formula
a = 4 cm, b = 5 cm, c =5 cm
s = (a +b+c)/2 = (4 +5+5)/2 =7 cm
= 2√21 = 2 × 4.58 = 9.16 cm²
Therefore area of ABCD = Area of ABC + Area of ADC = 6 +9.16 = 15.16 cm²
NCERT Solutions for Class 9 Maths exercise 12.2 Chapter 12 Heron’s Formula
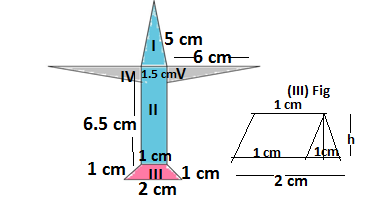
Q3.Radha made a picture of an aeroplane with the help of a coloured paper as shown in fig,12.5. Find the total area of the paper used.
Ans. Figure (I) is a isoscell triangular and (II) is rectangular section , the sides of triangle (I) are 5 cm,5cm and 1 cm
Fig 12.5 is following
Now let’s find the area of (I) by applying the Heron’s formula
a = 5 cm, b = 5 cm, c =1 cm
s = (a +b+c)/2 = (5 +5+1)/2 =5.5 cm
= √ (5.5×0.5 × 0.5× 4.5)= 1/100 √(55×5×5×45 =1/100√(11 ×5 ×5×5×9 ×5) =75√11/100=75×3.32/100 =249/100 =2.49 cm²
Figure (II) is a rectangle of the sides 1 cn and 6.5 cm ,area of figure (II) = 1 ×6.5 = 6.5 cm²
Figure (III) is a trapizium with parallel sides 1 cm and 2 cm, which contains a parallelogram with each sides 1 cm and and anequilateral triangle with each sides 1 cm
Area of figure (III) =1/2(sum of parallel sides)× height
Hight of the trapizium = Height of triangle = h
Area of triangular section = a²√3/4 = 1²√3/4= √3/4 cm ²
Area of Δ = 1/2(Base)× Height = 1/2 (1)h =h/2
h/2 = √3/4 ⇒ h = √3/2 cm
Therefore ar (III) = (1/2)(1+2) ×√3/2 = 3√3/4 = (3 ×1.732)/4 ≈1.3cm²
Figure (IV) and (V) are right triangles
Area of figure (IV) = Area of figure (V) = (1/2 )×6×1.5 = 4.5 cm²
Area of aeroplane = 2.49 + 6.5 +1.3 +4.5 +4.5 =19.29≈19.3 cm²
NCERT Solutions for Class 9 Maths exercise 12.2 Chapter 12 Heron’s Formula
Q4. Triangle and parallelogram have the same base and the same area. If the sides of the triangle are 26 cm, 28 cm, and 30 cm and the parallelogram stands on the base 28 cm, find the height of the parallelogram.
Ans. We are given the sides of a ΔDCE, DC = 28 cm, EC = 26 cm, DE = 30 cm
Also given EC = 28 cm common base of triangle DCE and quadrilateral ABCD and
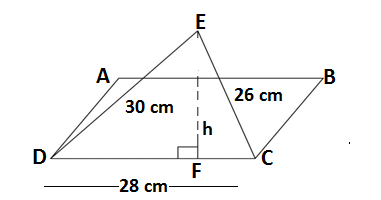
area of ΔDCE = ar ABCD(parallelogram)
√[s(s -a)(s-b)(s-c)] = (1/2) Base ×Height
s = (a +b+ c)/2 = (26 + 28 +30)/2 = 84/2 = 42 cm
√[42(42 -26)(42-28)(42-30)] = 28 ×h
28 ×h = √[42×16×14×12]
28h = √[3×7×2×16×2×7×2×2×3]
28 h = 3 ×4×2×2×7 =336
h = 12
Hence height of parallelogram is 12 cm
NCERT Solutions for Class 9 Maths exercise 12.2 Chapter 12 Heron’s Formula
Q5.A rhombus field has green grass for 18 cows to graze.If each sides of rhombus is 30 m and longer diagonal is 48 m.How much area of grass field will each cow be getting ?
Ans. We are given a rhombus ABCD ,whose each side is 30 cm and longer diagonal BD = 48 m
In ΔBCD,we have BC =30 m, CD = 30 m and BD = 48 m
ar Δ = √[s(s -a)(s-b)(s-c)]
s = (a +b+ c)/2 = (30 + 30 +48)/2 =10 8/2 = 54 m
ar ΔBCD = √[54(54 -30)(54-30)(54-48)]
= √[54×24×24×6] =√[6×9×6×4×6×4×6] = 6×6×4×3 =432
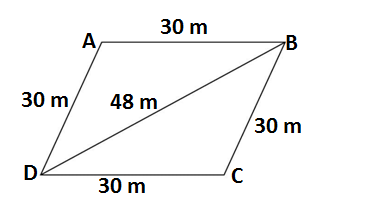
ar ABCD = 2ar ΔBCD ( Diagonal bisect the parallelogram in two equal parts)
= 2 × 432 = 864 m
The number of cows grazing in the field = 18
Therefore each cow be getting the area to graze in the field = 864/18 =48 m²
NCERT Solutions for Class 9 Maths exercise 12.2 Chapter 12 Heron’s Formula
Q6. An umbrella is made by stitching 10 triangular pieces of cloths of two different colours (see fig.12.16) , each piece measuring 20 cm,50 cm and 50 cm. How much cloth of each colour is required for the umbrella.
Ans. It is given to us that an umbrella is made of 10 triangular pieces of the sizze 20 cm,50 cm and 50 cm
ar Δ = √[s(s -a)(s-b)(s-c)]
s = (a +b+ c)/2 = (20 + 50 +50)/2 =120/2 = 60 m
ar Δ = √[60(60 -20)(60-50)(60-50)]
=√[60×40×10×10]=√(6×10×4×10×10×10) = 2×10×10√6 =200√6
Area of each triangular piece is = 200√6 cm²
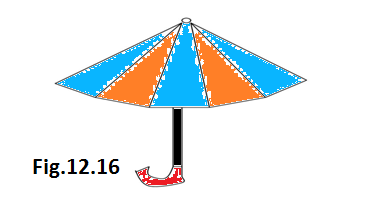
There are 10 triangular pieces in which 5 pieces are of the same colour a 5 are of another colour
Therefore cloth of each colour is required for the umbrella = 5× 200√6 =1000√6 cm²
NCERT Solutions for Class 9 Maths exercise 12.2 Chapter 12 Heron’s Formula
Q7.A kite in the shape of a square with a diagonal 32 cm and an isosceles triangle of base 8 cm and sides 6 cm each is to be made of three different shades as shown in Fig. 12.17. How much paper of each shade has been used in it?
Ans. We are given a kite of the shape of a square with 32 cm diagonal
(I )shade is a an isosceles triangle of the height 32/2 = 16 cm and base 32 cm
∴Area of I shade = (1/2) ×32 ×16 = 256 cm²
Area of II shade = Area of I shade (diagonal of the square divides the square in two equal parts)
Area of II shade = 256 cm²
III shade is an isisceles triangle of the base 8 cm and sides 6 cm,6 cm
ar Δ = √[s(s -a)(s-b)(s-c)]
s = (a +b+ c)/2 = (6 + 6+8)/2 =20/2 = 10 cm²
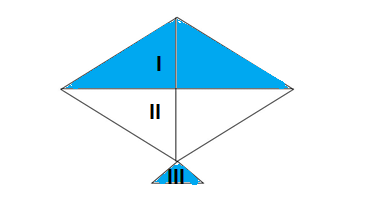
ar (III) = √[10(10 -6)(10-6)(10-8)]
= √[5×2×4×4×2] =8√5 cm²
NCERT Solutions for Class 9 Maths exercise 12.2 Chapter 12 Heron’s Formula
Q8.A floral design on a floor is made up of 16 tiles which are triangular, the sides of the triangle being 9 cm, 28 cm and 35 cm (see the fig.12.18) Find the cost of polishing the tiles at the rate of 50p per cm2 .
Ans. The given triangular tiles are 16, with sides 9 cm,28 cm and 35 cm
Area of a tile is computed by the Heron’s formula
ar Δ = √[s(s -a)(s-b)(s-c)]
s = (a +b+ c)/2 = (9 + 28+35)/2 =72/2 = 36 cm²
=√[36(36 -9)(36-28)(36-35)]
=√[36×27×8×1]= =√[36×9×3×4×2]=6×3×2√6 = 36√6 =36×2.449≈88.2cm²
Therefore area of 16 tiles = 16× 88.2 =1411.2 cm²
The rate of polishing = 50 p/cm² = Rs 0.50 /cm²

Hence cost of polishing the tiles are = 0.50 × 1411.2 = Rs 705.6
NCERT Solutions for Class 9 Maths exercise 12.2 Chapter 12 Heron’s Formula
Q9.A field is in the shape of a trapezium whose parallel sides are 25 m and 10 m. The non-parallel sides are 14 m and 13 m. Find the area of the field.
Ans. We are given a trapizium ABCD in which parallel sides are 25 m and 10 m and non-parallel sides are 14 m and 13 m
Drawing CE parallel to AD and CF ⊥ BE
We get a parallelogram ADCE and ΔBCE
Considering the ΔBCE in which BE = AB -AE = AB – DC= 25 – 10 = 15 m
Applyig the Heron’s formula for the area of ΔBCE
ar Δ = √[s(s -a)(s-b)(s-c)]
s = (a +b+ c)/2 = (13 + 14+15)/2 =42/2 = 21 cm
ar Δ BCE = √[21(21 -13)(21-14)(21-15)]
= √[21×8×7×6]=√[7×3×4×2×7×2×3] =7×2×3×2 = 84 m²
We also can get area of Δ BCE = (1/2) ×BE ×CF = (1/2)×15 CF
(1/2)×15 CF = 84
CF = (2×84)/15 =11.2 m
Since ABCD is a trapizium
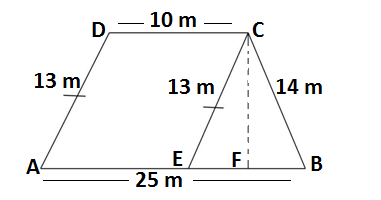
Area of a trapizium = (1/2) [10 + 25]×11.2 =(1/2)×35×11.2 = 196 m²
Hence area of the field is 196 cm²
You can compensate us by donating any amount of money for our survival
Our Paytm NO 9891436286
NCERT Solutions of class 9 science
CBSE Class 9-Question paper of science 2020 with solutions
CBSE Class 9-Sample paper of science
CBSE Class 9-Unsolved question paper of science 2019
NCERT Solutions of class 10 maths
CBSE Class 10-Question paper of maths 2021 with solutions
CBSE Class 10-Half yearly question paper of maths 2020 with solutions
CBSE Class 10 -Question paper of maths 2020 with solutions
CBSE Class 10-Question paper of maths 2019 with solutions
NCERT solutions of class 10 science
Solutions of class 10 last years Science question papers
CBSE Class 10 – Question paper of science 2020 with solutions
CBSE class 10 -Latest sample paper of science
NCERT solutions of class 11 maths
| Chapter 1-Sets | Chapter 9-Sequences and Series |
| Chapter 2- Relations and functions | Chapter 10- Straight Lines |
| Chapter 3- Trigonometry | Chapter 11-Conic Sections |
| Chapter 4-Principle of mathematical induction | Chapter 12-Introduction to three Dimensional Geometry |
| Chapter 5-Complex numbers | Chapter 13- Limits and Derivatives |
| Chapter 6- Linear Inequalities | Chapter 14-Mathematical Reasoning |
| Chapter 7- Permutations and Combinations | Chapter 15- Statistics |
| Chapter 8- Binomial Theorem | Chapter 16- Probability |
CBSE Class 11-Question paper of maths 2015
CBSE Class 11 – Second unit test of maths 2021 with solutions
NCERT solutions of class 12 maths
| Chapter 1-Relations and Functions | Chapter 9-Differential Equations |
| Chapter 2-Inverse Trigonometric Functions | Chapter 10-Vector Algebra |
| Chapter 3-Matrices | Chapter 11 – Three Dimensional Geometry |
| Chapter 4-Determinants | Chapter 12-Linear Programming |
| Chapter 5- Continuity and Differentiability | Chapter 13-Probability |
| Chapter 6- Application of Derivation | CBSE Class 12- Question paper of maths 2021 with solutions |
| Chapter 7- Integrals | |
| Chapter 8-Application of Integrals |
50 important science questions for cbse 10 class
Chemical properties of acid and base
Chemistry viva voce questions & answers
Chemistry practical based questions
Solutions of previous years science & maths question papers
Solutions of class 10 Science question paper 2019 CBSE
Solutions of class 10 CBSE maths question paper 2020
CBSE Class 10 science question paper 2020 SET -3 solutions
Download e-book of Science NCERT 634 questions with solutions for Polytechnic and NDA entrance exams