NCERT Solutions Class 9 Maths exercise 7.2 of the chapter 7-Triangles
NCERT Solutions Class 9 Maths exercise 7.2 of the chapter 7-Triangles is based on the solutions of the questions related to congruency of triangles.All questions of the exercise 7.2 -Triangles are solved by an expert of CBSE Maths.All students of class 9 are required to go through each solutions for clearing their concept on Triangles.You can study here science and maths NCERT solutions and notes of each chapters from class 9-12, sample papers, solutions of previous year’s question papers,solutions of important questions of science and maths ,articles on science and maths,government entrance exams and other competitive entrance exams and online jobs.
Click for online shopping
Future Study Point.Deal: Cloths, Laptops, Computers, Mobiles, Shoes etc
You can also study NCERT Solutions
NCERT Solutions of Class 9 Science : Chapter 1 to Chapter 15
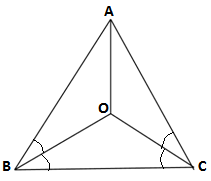
Q1.In an isosceles triangle ABC, with AB = AC, the bisector of angle ∠B and ∠C, intersect each other at O. Join A to O. Show that:
(i) OB = OC (ii) AO bisects ∠A
Ans.
GIVEN: In ΔABC
AB = AC
BO is bisector of ∠B and CO is bisector of ∠C
TO PROVE:(i) OB = OC (ii) AO bisects ∠A
PROOF:In ΔABC
AB = AC (given)
∠ABC = ∠ACB (angles opposite to equal sides)
It is given to us that BO is bisector of ∠B and CO is bisector of ∠C
1/2(∠ABC) = 1/2(∠ACB)
∠OBC = ∠OCB
OB = OC (sides opposite to equal angles)
Hence proved
(ii) OB = OC (proved above)
AO = AO (common)
AB = AC (given)
ΔABO ≅ΔACO (SSS rule)
∠OAB = ∠OCA (by CPCT)
Therefore AO is the bisector of ∠A.
Hence proved
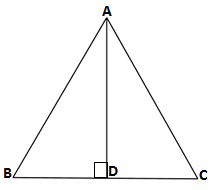
Q2. In ΔABC , AD is perpendicular bisector of BC (see the given figure). Show that ΔABC is an isoscles triangle in which AB = AC.
Ans.
GIVEN: In ΔABC , AD is perpendicular bisector of BC
∴ BD = DC
∠ADB = ∠ADC = 90°
TO PROVE: AB = AC
PROOF: In ΔABD and ΔACD
∠ADB = ∠ADC = 90°(given)
AD = AD (common)
BD = DC(given)
ΔABD ≅ ΔACD (SAS rule)
AB = AC(by CPCT)
Hence ΔABC is an isosceles triangle in which AB = AC
Q3. ABC is an isoscles triangle in which altitudes BE and CF are drawn to equal sides AC and AB respectively (see the given figure).Show that these altitudes are equal.
Ans.
GIVEN: ΔABC in which AC = AB
CF ⊥ AB and CE ⊥ AC
∴∠AFC = ∠AEB = 90°
TO PROVE: CF = BE
PROOF: AC = AB (given)
∠AFC = ∠AEB = 90°(given)
∠A = ∠A (common)
ΔAEB ≅ AFC (AAS rule)
BE = CF(by CPCT)
Hence proved
Q4.ABC is a triangle in which altitudes BE and CF to sides AC and AB are equal (see the given figure). Show that.
(i) ΔABE ≅ ACF
(ii)AB = AC i.e ABC is an isosceles triangle
Ans.
GIVEN: ΔABC in which BE = CF
CF ⊥ AB and CE ⊥ AC
∴∠AFC = ∠AEB = 90°
TO PROVE: AC = AB
(i) PROOF:BE = CF (given)
∠AFC = ∠AEB = 90°(given)
∠A = ∠A (common)
ΔAEB ≅ AFC (AAS rule)
(ii) AC = AB (by CPCT)
Hence proved
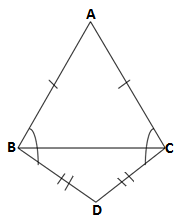
Q5. ABC and DBC are two isosceles triangles on the same base BC (see the given figure). Show that ∠ABD = ∠ACD.
Ans.
GIVEN: ΔABC in which AB = AC
ΔDBC in which BD = DC
TO PROVE: ∠ABD = ∠ACD
PROOF: In Δ ABC
AB = AC (given)
∠ABC = ∠ACB ….(i) (angles opposite to equal sides)
BD = DC
∠DBC = ∠DCB….(ii) (angles opposite to equal sides)
Adding equation (i) and (ii)
∠ABC + ∠DBC = ∠ACB + ∠DCB
∠ABD = ∠ACD
Hence proved
Q6. ΔABC is an isosceles triangle in which AB = AC, side BA is produced to D such that AD = AB(see the given figure). Show that ∠BCD is a right angle.
Ans.
GIVEN:AB = AC
AD = AB
TO PROVE: ∠BCD = 90°
PROOF : AB = AC (given)
∠ABC = ∠ACB (angles opposite to equal sides)
Applying the angle sum property in ΔABC
∠ABC + ∠BAC + ∠ACB = 180°
∠BAC = 180°- (∠ABC + ∠ACB)
∠BAC and ∠DAC are lienear pair
So, ∠BAC + ∠DAC = 180°
180°- (∠ABC + ∠ACB) + ∠DAC = 180°
∠DAC = ∠ABC + ∠ACB =∠ACB + ∠ACB =2∠ACB
∠ACB = 1/2 ∠DAC…..(i)
AD = AC (given)
∠ADC = ∠ACD (angles opposite to equal sides)
Applying the angle sum property in ΔABC
∠ADC + ∠ACD + ∠DAC = 180°
∠ACD + ∠ACD + ∠DAC = 180°
2∠ACD + ∠DAC = 180°
∠DAC = 180° – 2∠ACD
∠ACD = 90° – 1/2 ∠DAC……..(ii)
Adding equation (i) and (ii)
∠ACB + ∠ACD = 1/2 ∠DAC+ 90° – 1/2 ∠DAC
∠BCD = 90°
Hence ΔBCD is right triangle
Q7. ABC is a right triangle in which ∠A = 90° and AB = AC. Find ∠B and ∠C.
Ans.
We are given triagle ABC in which
∠A = 90°
AB = AC
∠B = ∠C (angles opposite to equal sides)
∠B + ∠C + ∠A = 180°
∠B + ∠B +90° = 180°
2∠B + 90° = 180°
2∠B = 90°
∠B = 45°
∠C = 45°
Hence both of the angles ∠B and ∠C are of 45°
Q8. Show that the angles of an equilateral triangle are 60° each.
Ans.
We are given that ΔABC is an equilateral triangle
AB = AC (sides of equilateral triangle)
∠C = ∠B …..(i)(angles opposite to equal sides)
BC = AC (sides of equilateral triangle)
∠B = ∠A …..(ii)(angles opposite to equal sides)
From equation (i) and (ii)
∠A = ∠B = ∠C
Applying angle sum property of the triangle in ΔABC
∠A +∠B +∠C = 180°
∠A + ∠A + ∠A = 180°
3∠A = 180°
∠A = 180°/3
∠A = 60°
∴∠A = ∠B =∠C=60°
Therefore angles of an equilateral triangle are 60° each.
You can compensate us by donating any amount of money for our survival
Our Paytm NO 9891436286
Paytm number 9891436286
The money collected by us will be used for the education of poor students who leaves their study because of a lack of money.
NCERT Solutions of Science and Maths for Class 9,10,11 and 12
NCERT Solutions for class 9 maths
NCERT Solutions for class 9 science
NCERT Solutions for class 10 maths
CBSE Class 10-Question paper of maths 2021 with solutions
CBSE Class 10-Half yearly question paper of maths 2020 with solutions
CBSE Class 10 -Question paper of maths 2020 with solutions
CBSE Class 10-Question paper of maths 2019 with solutions
NCERT Solutions for Class 10 Science
NCERT Solutions for class 11 maths
| Chapter 1-Sets | Chapter 9-Sequences and Series |
| Chapter 2- Relations and functions | Chapter 10- Straight Lines |
| Chapter 3- Trigonometry | Chapter 11-Conic Sections |
| Chapter 4-Principle of mathematical induction | Chapter 12-Introduction to three Dimensional Geometry |
| Chapter 5-Complex numbers | Chapter 13- Limits and Derivatives |
| Chapter 6- Linear Inequalities | Chapter 14-Mathematical Reasoning |
| Chapter 7- Permutations and Combinations | Chapter 15- Statistics |
| Chapter 8- Binomial Theorem | Chapter 16- Probability |
CBSE Class 11-Question paper of maths 2015
CBSE Class 11 – Second unit test of maths 2021 with solutions
NCERT solutions for class 12 maths
| Chapter 1-Relations and Functions | Chapter 9-Differential Equations |
| Chapter 2-Inverse Trigonometric Functions | Chapter 10-Vector Algebra |
| Chapter 3-Matrices | Chapter 11 – Three Dimensional Geometry |
| Chapter 4-Determinants | Chapter 12-Linear Programming |
| Chapter 5- Continuity and Differentiability | Chapter 13-Probability |
| Chapter 6- Application of Derivation | CBSE Class 12- Question paper of maths 2021 with solutions |
| Chapter 7- Integrals | |
| Chapter 8-Application of Integrals |
Class 12 Solutions of Maths Latest Sample Paper Published by CBSE for 2021-22 Term 2
Class 12 Maths Important Questions-Application of Integrals
Solutions of Class 12 Maths Question Paper of Preboard -2 Exam Term-2 CBSE Board 2021-22
Solutions of class 12 maths question paper 2021 preboard exam CBSE Solution